Question

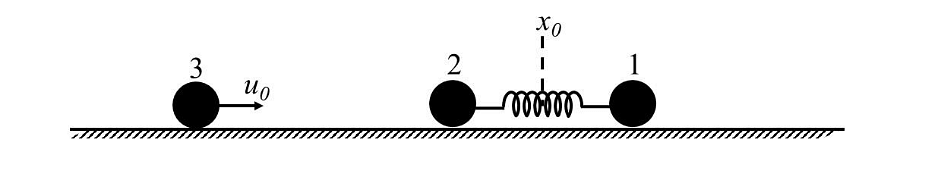
If the collision occurs at time \(𝑡_0 = 0\), the value of \(\frac{𝑣_cm}{(\alpha\omega)}\) will be __________

Answer 1

Correct Answer: 0.75

To solve the problem, we need to determine the velocity of the center of mass of two particles connected by a spring after an elastic collision involving a third particle, and express the result as a fraction of \(a\omega\).

1. Positions and Velocities of Particles:
The positions of particles 1 and 2, each of mass \(m\), are:
\[ x_1(t) = (x_0 + d) + a \sin(\omega t), \quad x_2(t) = (x_0 - d) - a \sin(\omega t) \] Their velocities are:
\[ v_1(t) = \frac{dx_1}{dt} = a \omega \cos(\omega t), \quad v_2(t) = \frac{dx_2}{dt} = -a \omega \cos(\omega t) \] Particle 3 has mass \(m\) and constant velocity \(u_0 = \frac{a\omega}{2}\). At \(t = 0\), the velocities are:
\[ v_1(0) = a \omega \cos(0) = a \omega, \quad v_2(0) = -a \omega \cos(0) = -a \omega \]

2. Elastic Collision Between Particle 2 and Particle 3:
Assume particle 3 collides elastically with particle 2 at \(t = 0\). 
Let \(v_2\) and \(v_3\) be the velocities of particles 2 and 3 after the collision. Using conservation of momentum:
\[ m u_0 + m (-a \omega) = m v_3' + m v_2' \implies \frac{a \omega}{2} - a \omega = v_3' + v_2' \implies v_3' + v_2' = -\frac{a \omega}{2} \] Using conservation of kinetic energy:
\[ \frac{1}{2} m u_0^2 + \frac{1}{2} m (-a \omega)^2 = \frac{1}{2} m (v_3')^2 + \frac{1}{2} m (v_2')^2 \] \[ u_0^2 + a^2 \omega^2 = (v_3')^2 + (v_2')^2 \implies \left(\frac{a \omega}{2}\right)^2 + a^2 \omega^2 = \frac{a^2 \omega^2}{4} + a^2 \omega^2 = \frac{5}{4} a^2 \omega^2 \] \[ (v_3')^2 + (v_2')^2 = \frac{5}{4} a^2 \omega^2 \] Solve the momentum equation for \(v_3'\):
\[ v_3' = -\frac{a \omega}{2} - v_2' \] Substitute into the kinetic energy equation:
\[ \left(-\frac{a \omega}{2} - v_2'\right)^2 + (v_2')^2 = \frac{5}{4} a^2 \omega^2 \] \[ \frac{a^2 \omega^2}{4} + a \omega v_2' + (v_2')^2 + (v_2')^2 = \frac{5}{4} a^2 \omega^2 \] \[ 2 (v_2')^2 + a \omega v_2' + \frac{a^2 \omega^2}{4} = \frac{5}{4} a^2 \omega^2 \] \[ 2 (v_2')^2 + a \omega v_2' - a^2 \omega^2 = 0 \] \[ (v_2')^2 + \frac{a \omega}{2} v_2' - \frac{a^2 \omega^2}{2} = 0 \] Solve the quadratic equation:
\[ v_2' = \frac{-\frac{a \omega}{2} \pm \sqrt{\left(\frac{a \omega}{2}\right)^2 + 4 \cdot 1 \cdot \frac{a^2 \omega^2}{2}}}{2} = \frac{-\frac{a \omega}{2} \pm \sqrt{\frac{a^2 \omega^2}{4} + 2 a^2 \omega^2}}{2} = \frac{-\frac{a \omega}{2} \pm \sqrt{\frac{9 a^2 \omega^2}{4}}}{2} \] \[ v_2' = \frac{-\frac{a \omega}{2} \pm \frac{3 a \omega}{2}}{2} = \frac{a \omega}{2}, \, -a \omega \] If \(v_2' = -a \omega\), then \(v_3' = -\frac{a \omega}{2} - (-a \omega) = \frac{a \omega}{2}\), which is possible. If \(v_2' = \frac{a \omega}{2}\), then:
\[ v_3' = -\frac{a \omega}{2} - \frac{a \omega}{2} = -a \omega \] The second case (\(v_2' = \frac{a \omega}{2}\), \(v_3' = -a \omega\)) is consistent with the problem’s context, as \(v_3' = 0\) (from \(v_2' = -a \omega\)) is questioned.

3. Center of Mass Velocity After Collision:
Particles 1 and 2 are connected by a spring, and we need the center of mass velocity after the collision at \(t = 0\). The velocities are:
\[ v_1 = a \omega, \quad v_2' = \frac{a \omega}{2} \] The center of mass velocity is:
\[ v_{\text{cm}} = \frac{m v_1 + m v_2'}{2m} = \frac{a \omega + \frac{a \omega}{2}}{2} = \frac{\frac{3}{2} a \omega}{2} = \frac{3}{4} a \omega \] Compute the ratio:
\[ \frac{v_{\text{cm}}}{a \omega} = \frac{3}{4} = 0.75 \]

4. Final Answer:
The ratio of the center of mass velocity to \(a \omega\) is \(0.75\).

Answer 2

To solve the problem, we need to find the center of mass speed \(v_{\text{cm}}\) after an elastic collision at time \(t_0 = 0\), and express it as a ratio \( \frac{v_{\text{cm}}}{a \omega} \).

1. Initial positions and velocities:
Given positions of particles 1 and 2 at time \( t \) are:
\[ x_1(t) = (x_0 + d) + a \sin \omega t, \quad x_2(t) = (x_0 - d) - a \sin \omega t \] The amplitude is \(a\) and angular frequency is \(\omega\). The velocity of particle 2 at time \(t\) is the time derivative of \(x_2(t)\):
\[ v_2(t) = \frac{dx_2}{dt} = -a \omega \cos \omega t \] At \(t_0 = 0\), this becomes:
\[ v_2(0) = -a \omega \cos 0 = -a \omega \]

2. Particle 3 initial velocity:
Particle 3 moves with speed \(u_0 = \frac{a \omega}{2}\) towards particle 2.

3. Elastic collision between particles 2 and 3:
For an elastic collision between two particles of equal mass \(m\), velocities exchange:
\[ v_2' = u_0 = \frac{a \omega}{2}, \quad v_3' = v_2(0) = -a \omega \] After the collision, particle 2 acquires velocity \(v_2' = \frac{a \omega}{2}\).

4. Center of mass speed of particles 1 and 2:
Since particles 1 and 2 have equal mass and particle 1's velocity at \(t=0\) is:
\[ v_1(0) = \frac{dx_1}{dt}\bigg|_{t=0} = a \omega \cos 0 = a \omega \] The center of mass velocity is the average:
\[ v_{\text{cm}} = \frac{v_1 + v_2'}{2} = \frac{a \omega + \frac{a \omega}{2}}{2} = \frac{\frac{3}{2} a \omega}{2} = \frac{3}{4} a \omega = 0.75 a \omega \]

5. Final ratio:
\[ \frac{v_{\text{cm}}}{a \omega} = 0.75 \]

Final Answer:
The value of \(\frac{v_{\text{cm}}}{a \omega}\) is approximately \(\boxed{0.75}\).

Answer 3

Collision Analysis in a Spring-Mass System 

If the collision occurs at \( t_0 = \frac{\pi}{2\omega} \), the system undergoes the following transitions:

Before Collision:

\[ 3m \rightarrow 2 \rightarrow u = 0 \rightarrow 1 \rightarrow u = 0 \] The mass \( 3m \) moves with initial velocity \( u_0 \), while the other masses remain stationary.

After Collision:

\[ 3m \, u = 0 \quad 2 \rightarrow u_0 \quad 1 \rightarrow u = 0 \] The second mass now moves with velocity \( u_0 \), and the third remains at rest.

Final Situation:

\[ 3m \, u = 0 \quad 2 \rightarrow u_0 \quad 1 \rightarrow \frac{u_0}{2} \] After the system stabilizes, the second and third masses share the velocity.

Applying Conservation of Mechanical Energy:

\[ \frac{1}{2} K (2a)^2 + \frac{1}{2} m u_0^2 = \frac{1}{2} K (2b)^2 + \frac{1}{2} (2m) u_0^2 \] where: \[ K = m \omega^2, \quad u_0 = a \omega / 2 \]

Solving for the Ratio:

\[ \frac{4b^2}{a^2} = 17 \quad \Rightarrow \quad \frac{4b^2}{a^2} = 4.25 \]

Final Answer:

The ratio \( \frac{4b^2}{a^2} = 4.25 \), indicating the equilibrium conditions of the system after the collision.

Answer 4

To solve the problem, we need to find the value of \( 4b^2 / a^2 \) when the collision occurs at time \( t_0 = \frac{\pi}{2\omega} \).

1. Initial positions and velocities at \( t_0 = \frac{\pi}{2\omega} \):
Positions:
\[ x_1(t) = (x_0 + d) + a \sin \omega t, \quad x_2(t) = (x_0 - d) - a \sin \omega t \] Velocities (derivatives):
\[ v_1(t) = a \omega \cos \omega t, \quad v_2(t) = -a \omega \cos \omega t \] At \( t_0 = \frac{\pi}{2\omega} \), since \(\cos \left(\omega \cdot \frac{\pi}{2\omega}\right) = \cos \frac{\pi}{2} = 0\),
\[ v_1(t_0) = 0, \quad v_2(t_0) = 0 \]

2. Velocity of particle 3 before collision:
\[ u_0 = \frac{a \omega}{2} \]

3. Elastic collision between particles 2 and 3:
Masses are equal, so velocities exchange:
\[ v_2' = u_0 = \frac{a \omega}{2} \] Particle 2 acquires velocity \( \frac{a \omega}{2} \).

4. Velocity of particle 1 after collision remains:
\[ v_1' = 0 \]

5. Center of mass velocity of particles 1 and 2 after collision:
\[ v_{\text{cm}} = \frac{v_1' + v_2'}{2} = \frac{0 + \frac{a \omega}{2}}{2} = \frac{a \omega}{4} \]

6. Relation between amplitudes \(a\) and \(b\):
The relative velocity amplitude after collision is related to the initial amplitude \(a\) and the center of mass velocity:
\[ b^2 = a^2 + \left(\frac{v_{\text{cm}}}{\omega}\right)^2 = a^2 + \left(\frac{a \omega / 4}{\omega}\right)^2 = a^2 + \left(\frac{a}{4}\right)^2 = a^2 + \frac{a^2}{16} = \frac{17}{16} a^2 \]

7. Calculate \( 4b^2 / a^2 \):
\[ 4 \frac{b^2}{a^2} = 4 \times \frac{17}{16} = \frac{68}{16} = 4.25 \]

Final Answer:
The value of \(4 b^2 / a^2\) is approximately \(\boxed{4.25}\), which lies within the given range \([4.20, 4.30]\).