Let Q be the cube with the set of vertices {(x1, x2, x3) ∈ R3: x1, x2, x3 ∈ {0,1}}. Let F be the set of all twelve lines containing the diagonals of the six faces of cube Q. Let S be the set of all four lines containing the main diagonals of the cube Q; for instance, the line passing through the vertices (0,0,0) and (1,1,1) is in S. For lines l1 and l2, let d(l1,l2) denote the shortest distance between them. Then the maximum value of d(l1,l2) as l1 varies over f and l2 varies over S, is
Let Q be the cube with the set of vertices {(x1, x2, x3) ∈ R3: x1, x2, x3 ∈ {0,1}}. Let F be the set of all twelve lines containing the diagonals of the six faces of cube Q. Let S be the set of all four lines containing the main diagonals of the cube Q; for instance, the line passing through the vertices (0,0,0) and (1,1,1) is in S. For lines l1 and l2, let d(l1,l2) denote the shortest distance between them. Then the maximum value of d(l1,l2) as l1 varies over f and l2 varies over S, is
- \(\frac{1}{\sqrt6}\)
- \(\frac{1}{\sqrt8}\)
- \(\frac{1}{\sqrt3}\)
- \(\frac{1}{\sqrt{12}}\)
The Correct Option is A
Solution and Explanation
The correct option is (A):
The equation of the OD line is
\(\vec{r_1}=\vec{0}+\lambda(\hat{i}+\hat{j})\)
equation of diagonal BE is
\(\vec{r_1}=\hat{j}+\alpha(\hat{i}-\hat{j}+\hat{k})\)
S.D = \(\left | \frac{\hat{j}.(\hat{i}-\hat{j}+\hat{k})}{\sqrt{6}} \right |=\frac{1}{\sqrt6}\)
Top Questions on introduction to three dimensional geometry
- If the 4th, 10th, and 16th terms of a G.P. are \(x\), \(y\), and \(z\) respectively, then
- KCET - 2025
- Mathematics
- introduction to three dimensional geometry
- The number of diagonals that can be drawn in an octagon is:
- KCET - 2025
- Mathematics
- introduction to three dimensional geometry
- The equation of the line through the point \( (0, 1, 2) \) and perpendicular to the line \[ \frac{x - 1}{2} = \frac{y + 1}{3} = \frac{z - 1}{-2} \] is:
- KCET - 2025
- Mathematics
- introduction to three dimensional geometry
- Let \( \vec{a}, \vec{b}, \vec{c} \) be three vectors. The angle between \( \vec{a} \) and \( \vec{b} \) is \( 30^\circ \), the angle between \( \vec{a} \) and \( \vec{b} + \vec{c} \) is \( 45^\circ \). If \( |\vec{b}| = \sqrt{6} \) and \( |\vec{c}| = 2\sqrt{2} \), then \( |\vec{b} + \vec{c}| \) is:
- KEAM - 2024
- Mathematics
- introduction to three dimensional geometry
- Let \[ f(x) = \frac{|5 - x|(x + 5)}{\tan(x - 5)} \quad {for} \quad x \neq 5. \] Then \[ \lim_{x \to 5} f(x) { is equal to:} \]
- KEAM - 2024
- Mathematics
- introduction to three dimensional geometry
Questions Asked in JEE Advanced exam
Let $ a_0, a_1, ..., a_{23} $ be real numbers such that $$ \left(1 + \frac{2}{5}x \right)^{23} = \sum_{i=0}^{23} a_i x^i $$ for every real number $ x $. Let $ a_r $ be the largest among the numbers $ a_j $ for $ 0 \leq j \leq 23 $. Then the value of $ r $ is ________.
- JEE Advanced - 2025
- binomial expansion formula
- Consider the vectors $$ \vec{x} = \hat{i} + 2\hat{j} + 3\hat{k},\quad \vec{y} = 2\hat{i} + 3\hat{j} + \hat{k},\quad \vec{z} = 3\hat{i} + \hat{j} + 2\hat{k}. $$ For two distinct positive real numbers $ \alpha $ and $ \beta $, define $$ \vec{X} = \alpha \vec{x} + \beta \vec{y} - \vec{z},\quad \vec{Y} = \alpha \vec{y} + \beta \vec{z} - \vec{x},\quad \vec{Z} = \alpha \vec{z} + \beta \vec{x} - \vec{y}. $$ If the vectors $ \vec{X}, \vec{Y}, \vec{Z} $ lie in a plane, then the value of $ \alpha + \beta - 3 $ is ________.
Let $ y(x) $ be the solution of the differential equation $$ x^2 \frac{dy}{dx} + xy = x^2 + y^2, \quad x > \frac{1}{e}, $$ satisfying $ y(1) = 0 $. Then the value of $ 2 \cdot \frac{(y(e))^2}{y(e^2)} $ is ________.
- JEE Advanced - 2025
- Differential equations
The left and right compartments of a thermally isolated container of length $L$ are separated by a thermally conducting, movable piston of area $A$. The left and right compartments are filled with $\frac{3}{2}$ and 1 moles of an ideal gas, respectively. In the left compartment the piston is attached by a spring with spring constant $k$ and natural length $\frac{2L}{5}$. In thermodynamic equilibrium, the piston is at a distance $\frac{L}{2}$ from the left and right edges of the container as shown in the figure. Under the above conditions, if the pressure in the right compartment is $P = \frac{kL}{A} \alpha$, then the value of $\alpha$ is ____
- JEE Advanced - 2025
- Thermodynamics
- The total number of real solutions of the equation $$ \theta = \tan^{-1}(2 \tan \theta) - \frac{1}{2} \sin^{-1} \left( \frac{6 \tan \theta}{9 + \tan^2 \theta} \right) $$ is
(Here, the inverse trigonometric functions $ \sin^{-1} x $ and $ \tan^{-1} x $ assume values in $[-\frac{\pi}{2}, \frac{\pi}{2}]$ and $(-\frac{\pi}{2}, \frac{\pi}{2})$, respectively.)- JEE Advanced - 2025
- Inverse Trigonometric Functions
Concepts Used:
Three Dimensional Geometry
Mathematically, Geometry is one of the most important topics. The concepts of Geometry are derived w.r.t. the planes. So, Geometry is divided into three major categories based on its dimensions which are one-dimensional geometry, two-dimensional geometry, and three-dimensional geometry.
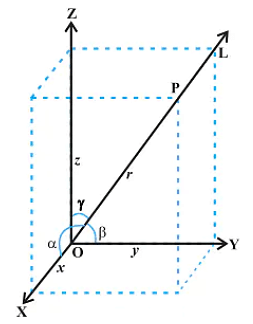
Direction Cosines and Direction Ratios of Line:
Consider a line L that is passing through the three-dimensional plane. Now, x,y and z are the axes of the plane and α,β, and γ are the three angles the line makes with these axes. These are commonly known as the direction angles of the plane. So, appropriately, we can say that cosα, cosβ, and cosγ are the direction cosines of the given line L.
