Let M=(aij), i,j∈{1,2,3}, be the 3×3 matrix such that aij=1 if j+1 is divisible by i,otherwise aij=0. Then which of the following statements is(are) true?
- M is invertible
- There exists a nonzero column matrix\(\begin{pmatrix}a_1\\a_2\\a_3\end{pmatrix}\) such that M\(\begin{pmatrix}a_1\\a_2\\a_3\end{pmatrix}\)=\(\begin{pmatrix}-a_1\\-a_2\\-a_3\end{pmatrix}\)
- The set {\({x\in R^3:MX=0}\)} \(\neq\) 0, where 0=\(\begin{pmatrix}0\\0\\0\end{pmatrix}\)
- The matrix ( M-2I) is invertible, where I is the 3×3 identity matrix
The Correct Option is B, C
Solution and Explanation
Given :
M = (aij), i, j ∈ {1, 2, 3},
aij = 1 if j + 1 is divisible by i, otherwise aij = 0
\(M=\begin{bmatrix} 1 & 1 & 1 \\ 1 & 0 & 1 \\ 0 & 1 & 0 \end{bmatrix}\)
|M| = 1(-1) - 1(-1)
= -1 + 1 = 0
So, M is not invertible
\(\begin{bmatrix} 1 & 1 & 1 \\ 1 & 0 & 1 \\ 0 & 1 & 0 \end{bmatrix}\begin{bmatrix} a_{1} \\ a_{2} \\ a_{3} \end{bmatrix}=\begin{bmatrix} -a_{1} \\ -a_{2} \\ -a_{3} \end{bmatrix}\)
\(\begin{bmatrix} a_1+ a_2 +a_3 \\ a_1+a_3 \\ a_2 \end{bmatrix}=\begin{bmatrix} -a_{1} \\ -a_{2} \\ -a_{3} \end{bmatrix}\)
There exists an infinite number of possible column matrices.
\(\begin{bmatrix} 1 & 1 & 1 \\ 1 & 0 & 1 \\ 0 & 1 & 0 \end{bmatrix}\begin{bmatrix} x \\ y \\ z \end{bmatrix}=\begin{bmatrix} 0 \\ 0 \\ 0 \end{bmatrix}\)
x + y + z = 0
⇒ x + z = 0
y = 0
So, this is possible only.
\(|M-2I|=\begin{bmatrix} -1 & 1 & 1 \\ 1 & -2 & 1 \\ 0 & 1 & -2 \end{bmatrix}\)
\(=-1(3)-1(-2-1)=-3+3=0\)
So, the correct options are (B) and (C).
Top Questions on Matrices
- Let \( A = \begin{bmatrix} 1 & -2 & -1 \\ 0 & 4 & -1 \\ -3 & 2 & 1 \end{bmatrix}, B = \begin{bmatrix} -5 \\ -2 \end{bmatrix}, C = [9 \ \ 7], \) which of the following is defined?
- If \[ \begin{bmatrix} 4 + x & x - 1 \\ -2 & 3 \end{bmatrix} \] is a singular matrix, then the value of \( x \) is:
If \[ A = \begin{bmatrix} 1 & 2 & 0 \\ -2 & -1 & -2 \\ 0 & -1 & 1 \end{bmatrix} \] then find \( A^{-1} \). Hence, solve the system of linear equations: \[ x - 2y = 10, \] \[ 2x - y - z = 8, \] \[ -2y + z = 7. \]
- Find x, y, z if
\[ \begin{bmatrix} 5 & 1 & 0 \\ 1 & 1 & 1 \end{bmatrix} \begin{bmatrix} 0 & 1 & -2 \\ 1 & -2 & 3 \\ -1 & 1 & 1 \end{bmatrix} \begin{bmatrix} x-1 \\ y+1 \\ 2z \end{bmatrix} = \begin{bmatrix} 2 \\ 1 \end{bmatrix}. \] - Solve the following equations by the method of inversion: \( 2x-y+z=1 \), \( x+2y+3z=8 \), \( 3x+y-4z=1 \).
Questions Asked in JEE Advanced exam
- Consider the vectors $$ \vec{x} = \hat{i} + 2\hat{j} + 3\hat{k},\quad \vec{y} = 2\hat{i} + 3\hat{j} + \hat{k},\quad \vec{z} = 3\hat{i} + \hat{j} + 2\hat{k}. $$ For two distinct positive real numbers $ \alpha $ and $ \beta $, define $$ \vec{X} = \alpha \vec{x} + \beta \vec{y} - \vec{z},\quad \vec{Y} = \alpha \vec{y} + \beta \vec{z} - \vec{x},\quad \vec{Z} = \alpha \vec{z} + \beta \vec{x} - \vec{y}. $$ If the vectors $ \vec{X}, \vec{Y}, \vec{Z} $ lie in a plane, then the value of $ \alpha + \beta - 3 $ is ________.
Let $ a_0, a_1, ..., a_{23} $ be real numbers such that $$ \left(1 + \frac{2}{5}x \right)^{23} = \sum_{i=0}^{23} a_i x^i $$ for every real number $ x $. Let $ a_r $ be the largest among the numbers $ a_j $ for $ 0 \leq j \leq 23 $. Then the value of $ r $ is ________.
- JEE Advanced - 2025
- binomial expansion formula
- The total number of real solutions of the equation $$ \theta = \tan^{-1}(2 \tan \theta) - \frac{1}{2} \sin^{-1} \left( \frac{6 \tan \theta}{9 + \tan^2 \theta} \right) $$ is
(Here, the inverse trigonometric functions $ \sin^{-1} x $ and $ \tan^{-1} x $ assume values in $[-\frac{\pi}{2}, \frac{\pi}{2}]$ and $(-\frac{\pi}{2}, \frac{\pi}{2})$, respectively.)- JEE Advanced - 2025
- Inverse Trigonometric Functions
A temperature difference can generate e.m.f. in some materials. Let $ S $ be the e.m.f. produced per unit temperature difference between the ends of a wire, $ \sigma $ the electrical conductivity and $ \kappa $ the thermal conductivity of the material of the wire. Taking $ M, L, T, I $ and $ K $ as dimensions of mass, length, time, current and temperature, respectively, the dimensional formula of the quantity $ Z = \frac{S^2 \sigma}{\kappa} $ is:
- JEE Advanced - 2025
- Dimensional Analysis
- Let $$ \alpha = \frac{1}{\sin 60^\circ \sin 61^\circ} + \frac{1}{\sin 62^\circ \sin 63^\circ} + \cdots + \frac{1}{\sin 118^\circ \sin 119^\circ}. $$ Then the value of $$ \left( \frac{\csc 1^\circ}{\alpha} \right)^2 $$ is \rule{1cm}{0.15mm}.
- JEE Advanced - 2025
- Some Applications of Trigonometry
Concepts Used:
Transpose of a Matrix
The matrix acquired by interchanging the rows and columns of the parent matrix is called the Transpose matrix. The transpose matrix is also defined as - “A Matrix which is formed by transposing all the rows of a given matrix into columns and vice-versa.”
The transpose matrix of A is represented by A’. It can be better understood by the given example:

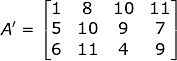
Now, in Matrix A, the number of rows was 4 and the number of columns was 3 but, on taking the transpose of A we acquired A’ having 3 rows and 4 columns. Consequently, the vertical Matrix gets converted into Horizontal Matrix.
Hence, we can say if the matrix before transposing was a vertical matrix, it will be transposed to a horizontal matrix and vice-versa.
Read More: Transpose of a Matrix