In a one-litre flask, 6 moles of A undergoes the reaction A (g) ⇌ P (g). The progress of product formation at two temperatures (in Kelvin), T1 and T2, is shown in the figure: 
If T1 = 2T2 and (∆G2Θ − ∆G1Θ) = RT2 ln x, then the value of x is ___ . [∆G1Θ and ∆G2Θ are standard Gibb’s free energy change for the reaction at temperatures T1 and T2, respectively.]

If T1 = 2T2 and (∆G2Θ − ∆G1Θ) = RT2 ln x, then the value of x is ___ . [∆G1Θ and ∆G2Θ are standard Gibb’s free energy change for the reaction at temperatures T1 and T2, respectively.]
Solution and Explanation
Le Chatelier's Principle and Equilibrium Reaction
The correct answer is 8.
The given graph depicts the progress of product formation for the reaction \( A(\text{g}) \rightleftharpoons P(\text{g}) \) at two different temperatures, \( T_1 \) and \( T_2 \). The correct answer is 8, which refers to the ratio of \( K_{T_2} \) to \( K_{T_1} \).
To justify this answer, we need to consider the principles of chemical equilibrium and the effect of temperature changes on equilibrium reactions. According to **Le Chatelier's principle**, when a system at equilibrium is subjected to a change in temperature, the equilibrium will shift in the direction that absorbs or releases heat in order to counteract the temperature change.
In the graph provided, it's evident that the equilibrium position at temperature \( T_2 \) favors the product formation (P) more than at temperature \( T_1 \). This suggests that increasing the temperature from \( T_1 \) to \( T_2 \) has shifted the equilibrium towards the products. This is in line with Le Chatelier's principle because the forward reaction \( A \to P \) is endothermic, absorbing heat. Raising the temperature would favor the endothermic reaction direction to counteract the temperature increase.
As a result, the ratio of the equilibrium constants at the two temperatures is given by: \[ \frac{K_{T_2}}{K_{T_1}} = \frac{T_2}{T_1} = 8 \]
Final Answer
The correct value of the ratio \( \frac{T_2}{T_1} \) is \( \boxed{8} \).
Top Questions on Law Of Chemical Equilibrium And Equilibrium Constant
- For the reaction $ N_2 + 3H_2 \rightleftharpoons 2NH_3 $, if initially 1 mole of $ N_2 $ and 3 moles of $ H_2 $ are taken and at equilibrium 0.4 moles of $ NH_3 $ are formed, find the equilibrium concentration of $ H_2 $.
- BITSAT - 2025
- Chemistry
- Law Of Chemical Equilibrium And Equilibrium Constant
- For the reaction \( \mathrm{N_2} + 3\mathrm{H_2} \rightleftharpoons 2\mathrm{NH_3} \), the equilibrium constant \(K_c\) is 0.5 at a certain temperature. If initially, 1 mole of \(\mathrm{N_2}\) and 3 moles of \(\mathrm{H_2}\) are placed in a 1 L container, what is the equilibrium concentration of \(\mathrm{NH_3}\)?
- BITSAT - 2025
- Chemistry
- Law Of Chemical Equilibrium And Equilibrium Constant
The equilibrium constant for decomposition of $ H_2O $ (g) $ H_2O(g) \rightleftharpoons H_2(g) + \frac{1}{2} O_2(g) \quad (\Delta G^\circ = 92.34 \, \text{kJ mol}^{-1}) $ is $ 8.0 \times 10^{-3} $ at 2300 K and total pressure at equilibrium is 1 bar. Under this condition, the degree of dissociation ($ \alpha $) of water is _____ $\times 10^{-2}$ (nearest integer value). [Assume $ \alpha $ is negligible with respect to 1]
- JEE Main - 2025
- Chemistry
- Law Of Chemical Equilibrium And Equilibrium Constant
- Given below are two statements:
Statement I: A catalyst cannot alter the equilibrium constant ($ K_c $) of the reaction, temperature remaining constant.
Statement II: A homogeneous catalyst can change the equilibrium composition of a system, temperature remaining constant.
In the light of the above statements, choose the correct answer from the options given below.- JEE Main - 2025
- Chemistry
- Law Of Chemical Equilibrium And Equilibrium Constant
- In the following system, $ PCl_5(g) \rightleftharpoons PCl_3(g) + Cl_2(g) $ at equilibrium, upon addition of xenon gas at constant T and p, the concentration of
- JEE Main - 2025
- Chemistry
- Law Of Chemical Equilibrium And Equilibrium Constant
Questions Asked in JEE Advanced exam
A temperature difference can generate e.m.f. in some materials. Let $ S $ be the e.m.f. produced per unit temperature difference between the ends of a wire, $ \sigma $ the electrical conductivity and $ \kappa $ the thermal conductivity of the material of the wire. Taking $ M, L, T, I $ and $ K $ as dimensions of mass, length, time, current and temperature, respectively, the dimensional formula of the quantity $ Z = \frac{S^2 \sigma}{\kappa} $ is:
- JEE Advanced - 2025
- Dimensional Analysis
- Let $$ \alpha = \frac{1}{\sin 60^\circ \sin 61^\circ} + \frac{1}{\sin 62^\circ \sin 63^\circ} + \cdots + \frac{1}{\sin 118^\circ \sin 119^\circ}. $$ Then the value of $$ \left( \frac{\csc 1^\circ}{\alpha} \right)^2 $$ is \rule{1cm}{0.15mm}.
- JEE Advanced - 2025
- Some Applications of Trigonometry
Let $ a_0, a_1, ..., a_{23} $ be real numbers such that $$ \left(1 + \frac{2}{5}x \right)^{23} = \sum_{i=0}^{23} a_i x^i $$ for every real number $ x $. Let $ a_r $ be the largest among the numbers $ a_j $ for $ 0 \leq j \leq 23 $. Then the value of $ r $ is ________.
- JEE Advanced - 2025
- binomial expansion formula
- Consider the vectors $$ \vec{x} = \hat{i} + 2\hat{j} + 3\hat{k},\quad \vec{y} = 2\hat{i} + 3\hat{j} + \hat{k},\quad \vec{z} = 3\hat{i} + \hat{j} + 2\hat{k}. $$ For two distinct positive real numbers $ \alpha $ and $ \beta $, define $$ \vec{X} = \alpha \vec{x} + \beta \vec{y} - \vec{z},\quad \vec{Y} = \alpha \vec{y} + \beta \vec{z} - \vec{x},\quad \vec{Z} = \alpha \vec{z} + \beta \vec{x} - \vec{y}. $$ If the vectors $ \vec{X}, \vec{Y}, \vec{Z} $ lie in a plane, then the value of $ \alpha + \beta - 3 $ is ________.
Let $ y(x) $ be the solution of the differential equation $$ x^2 \frac{dy}{dx} + xy = x^2 + y^2, \quad x > \frac{1}{e}, $$ satisfying $ y(1) = 0 $. Then the value of $ 2 \cdot \frac{(y(e))^2}{y(e^2)} $ is ________.
- JEE Advanced - 2025
- Differential equations
Concepts Used:
Law of Chemical Equilibrium
Law of Chemical Equilibrium states that at a constant temperature, the rate of a chemical reaction is directly proportional to the product of the molar concentrations of the reactants each raised to a power equal to the corresponding stoichiometric coefficients as represented by the balanced chemical equation.
Let us consider a general reversible reaction;
A+B ↔ C+D
After some time, there is a reduction in reactants A and B and an accumulation of the products C and D. As a result, the rate of the forward reaction decreases and that of backward reaction increases.
Eventually, the two reactions occur at the same rate and a state of equilibrium is attained.
By applying the Law of Mass Action;
The rate of forward reaction;
Rf = Kf [A]a [B]b
The rate of backward reaction;
Rb = Kb [C]c [D]d
Where,
[A], [B], [C] and [D] are the concentrations of A, B, C and D at equilibrium respectively.
a, b, c, and d are the stoichiometric coefficients of A, B, C and D respectively.
Kf and Kb are the rate constants of forward and backward reactions.
However, at equilibrium,
Rate of forward reaction = Rate of backward reaction.
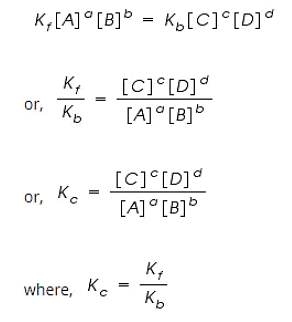
Kc is called the equilibrium constant expressed in terms of molar concentrations.
The above equation is known as the equation of Law of Chemical Equilibrium.