If two equal chords of a circle intersect within the circle, prove that the segments of one chord are equal to corresponding segments of the other chord.
Solution and Explanation
Let PQ and RS be two equal chords of a given circle and they are intersecting each other at point T.

Draw perpendiculars OV and OU on these chords.
In ∆OVT and ∆OUT,
OV = OU (Equal chords of a circle are equidistant from the centre)
∠OVT = ∠OUT (Each 90°)
OT = OT (Common)
∴ ∆OVT ≅ ∆OUT (RHS congruence rule)
∴ VT = UT (By CPCT) ... (1)
It is given that,
PQ = RS ... (2)
⇒ \(\frac{1}{2}\) PQ= \(\frac{1}{2}\) RS
⇒ PV = RU ... (3)
On adding equations (1) and (3), we obtain
PV + VT = RU + UT
⇒ PT = RT ... (4)
On subtracting equation (4) from equation (2), we obtain
PQ − PT = RS − RT
⇒ QT = ST ... (5)
Equations (4) and (5) indicate that the corresponding segments of chords PQ and RS are congruent to each other.
Top Questions on Perpendicular from the Centre to a Chord
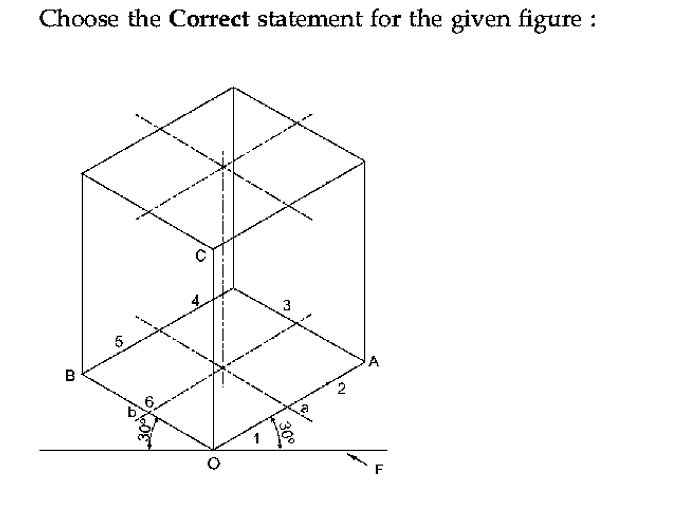
- Choose the Correct statement for the given figure:
- CUET (UG) - 2023
- Informatics Practices
- Perpendicular from the Centre to a Chord
- Identify the correct statements for the given figure :

(A) A triangular pyramid is resting on H.P. on its base.
(B) A triangular pyramid is resting on H.P. on its apex.
(C) Axis of pyramid is perpendicular to H.P.
(D) Axis of pyramid is parallel to H.P.
(E) Axis of pyramid is perpendicular to V.P.
Choose the correct answer from the options given below:
- CUET (UG) - 2023
- Engineering Graphics
- Perpendicular from the Centre to a Chord
- If two equal chords of a circle intersect within the circle, prove that the line joining the point of intersection to the centre makes equal angles with the chords.
- CBSE Class IX
- Mathematics
- Perpendicular from the Centre to a Chord
Questions Asked in CBSE Class IX exam
- Narrate two incidents that show how differences can be created, and also how they can be resolved. How can people change their attitudes?
- CBSE Class IX
- My childhood
- (i) His thoughts when he looks into the mirror
(ii) His thoughts when the snake is coiled around his arm- CBSE Class IX
- The snake and the mirror
(i) The kind of person the doctor is (money, possessions)
(ii) The kind of person he wants to be (appearance, ambition)- CBSE Class IX
- The snake and the mirror
- What are the main features of the mechanical teachers and the schoolrooms that Margie and Tommy have in the story?
- CBSE Class IX
- The fun they had
- What incidents during the Everest expedition show Santosh’s concern for her team-mates?
- CBSE Class IX
- Reach for the top