If in two circles, arcs of the same length subtend angles 60° and 75° at the centre, find the ratio of their radii.
Solution and Explanation
Let the radii of the two circles be r1 and r2 . Let an arc of length l subtend an angle of 60° at the centre of the circle of radiusr1, while let an arc of length l subtend an angle of 75° at the centre of the circle of radius r2
\(Now, \,60° = \frac{\pi}{3} \text{radian and\,75°} =\frac{\pi}{12}\,radian\)
We know that in a circle of radius r unit, if an arc of length l unit subtends an angle θ radian at the centre, then
\(θ=\frac{l}{r}\,or\,l=rθ\)
\(l=\frac{r_1{\pi}}{3}\,and\,l=\frac{r_25{\pi}}{12}\)
\(=\frac{r_1{\pi}}{3}\,and\,l=\frac{r_25{\pi}}{12}\)
\(r_1=\frac{r_25}{4}\)
\(\frac{r_1}{r_2}=\frac{5}{4}\)
Thus, the ratio of the radii is 5:4.
Top Questions on Trigonometric Functions
- Evaluate: $ \tan^{-1} \left[ 2 \sin \left( 2 \cos^{-1} \frac{\sqrt{3}}{2} \right) \right]$
- CBSE CLASS XII - 2025
- Mathematics
- Trigonometric Functions
- What is \( \tan \left( \frac{1}{2} \left( \tan^{-1} x + \tan^{-1} \frac{1}{x} \right) \right) \)?
- Bihar Board XII - 2025
- Mathematics
- Trigonometric Functions
- \(\int \frac{\cos 2x}{\cos x + \sin x} dx \)
- Bihar Board XII - 2025
- Mathematics
- Trigonometric Functions
- In \( \triangle ABC \), if \( a = 13 \), \( b = 14 \), and \( c = 15 \), then find the values of:
(i) \( \sec A \)
(ii) \( \csc \frac{A}{2} \)- Maharashtra Class XII - 2025
- Mathematics & Statistics
- Trigonometric Functions
- Solve for \( x \), \[ 2 \tan^{-1} x + \sin^{-1} \left( \frac{2x}{1 + x^2} \right) = 4\sqrt{3} \]
- CBSE CLASS XII - 2025
- Mathematics
- Trigonometric Functions
Questions Asked in CBSE Class XI exam
- The following three compound words end in -ship. What does each of them mean?
airship flagship lightship - CBSE Class XI
- We're not afraid to Die if we can all be together
- Write the resonance structures for SO3 , NO2 and NO3-
- CBSE Class XI
- Kossel-Lewis Approach to Chemical Bonding
- Two ideal gas thermometers A and B use oxygen and hydrogen respectively. The following observations are made :
Temperature Pressure thermometer A Pressure thermometer B Triple-point of water 1.250 × 10\(^5\) Pa 0.200 × 10\(^5\) Pa Normal melting point of sulphur 1.797× 10\(^5\) Pa 0.287 × 10\(^5\) Pa - What is the absolute temperature of normal melting point of sulphur as read by thermometers A and B?
- What do you think is the reason behind the slight difference in answers of thermometers A and B ? (The thermometers are not faulty). What further procedure is needed in the experiment to reduce the discrepancy between the two readings?
- CBSE Class XI
- Thermal Expansion
- List the steps taken by the captain
(i) to protect the ship when rough weather began.
(ii) to check the flooding of the water in the ship.- CBSE Class XI
- We're not afraid to Die if we can all be together
- Prove that. \(2sin^2 \frac{π}{6}+cosec^2 \frac{7π}{6}–cos^2 \frac{π}{3}=\frac{3}{2}\)
- CBSE Class XI
- Trigonometric Functions of Sum and Difference of Two Angles
Concepts Used:
Trigonometric Functions
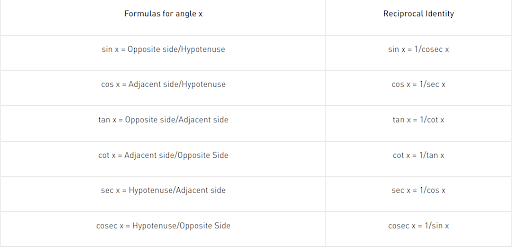
The relationship between the sides and angles of a right-angle triangle is described by trigonometry functions, sometimes known as circular functions. These trigonometric functions derive the relationship between the angles and sides of a triangle. In trigonometry, there are three primary functions of sine (sin), cosine (cos), tangent (tan). The other three main functions can be derived from the primary functions as cotangent (cot), secant (sec), and cosecant (cosec).
Six Basic Trigonometric Functions:
- Sine Function: The ratio between the length of the opposite side of the triangle to the length of the hypotenuse of the triangle.
sin x = a/h
- Cosine Function: The ratio between the length of the adjacent side of the triangle to the length of the hypotenuse of the triangle.
cos x = b/h
- Tangent Function: The ratio between the length of the opposite side of the triangle to the adjacent side length.
tan x = a/b
Tan x can also be represented as sin x/cos x
- Secant Function: The reciprocal of the cosine function.
sec x = 1/cosx = h/b
- Cosecant Function: The reciprocal of the sine function.
cosec x = 1/sinx = h/a
- Cotangent Function: The reciprocal of the tangent function.
cot x = 1/tan x = b/a
Formulas of Trigonometric Functions: