For the reaction, $X (s) \rightleftharpoons Y (s)+ Z (g)$, the plot of $\ln \frac{p_{ Z }}{p^{0}}$ versus $\frac{10^{4}}{T}$ is given below (in solid line), where $p_{ z }$ is the pressure (in bar) of the gas $Z$ at temperature $T$ and $p^0=1$ bar
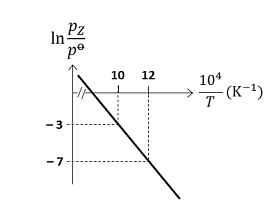
(Given, $\frac{ d (\ln K)}{ d \left(\frac{1}{T}\right)}=-\frac{\Delta H^{0}}{R}$, where the equilibrium constant, $K=\frac{p_{z}}{p^{0}}$ and the gas constant, $R=8.314 \,J \,K ^{-1} mol ^{-1}$ )
The value of $\Delta S^{0}$ (in $J\, K ^{-1} mol ^{-1}$ ) for the given reaction, at $1000\, K$ is ______
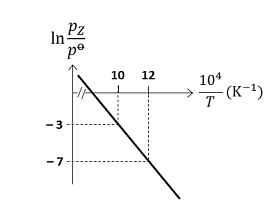
(Given, $\frac{ d (\ln K)}{ d \left(\frac{1}{T}\right)}=-\frac{\Delta H^{0}}{R}$, where the equilibrium constant, $K=\frac{p_{z}}{p^{0}}$ and the gas constant, $R=8.314 \,J \,K ^{-1} mol ^{-1}$ )
The value of $\Delta S^{0}$ (in $J\, K ^{-1} mol ^{-1}$ ) for the given reaction, at $1000\, K$ is ______
Correct Answer: 141.34
Solution and Explanation
The equation that relates the standard Gibbs free energy change (\(\Delta G\degree\)), the enthalpy change (\(\Delta H\degree\)), and the entropy change (\(\Delta S\degree\)) is:
\(\Delta G\degree=\Delta H\degree-T\Delta S\degree.....(i)\)
where T is the temperature in kelvin and R is the gas constant.
The equation that relates the equilibrium constant (K) and the standard Gibbs free energy change is:
\(\Delta G\degree=-RT\ In(K).....(ii)\)
We can combine these two equations to eliminate ΔG° and solve for K.
Given that \(K=\frac{p^2}{p\degree}\) and P° = 1 bar
\(ln(\frac{P}{1}) = \frac{-\Delta H\degree}{RT} + \frac{\Delta S\degree}{R}.....(iii)\)
The slope of the graph in the image is equal to \(\frac{-\Delta H\degree}{R}\).
Slope = \(10^4(\frac{Y_2-Y_1}{X_2-X_1})=10^4(\frac{-7+3}{12-10})=-2\times10^4\)
We can use the gas constant value (R = 8.314 J/mol*K) to find ΔH°.
\(-2\times10^4=\frac{\Delta H\degree}{R}=\gt2\times10^4\times8.314\)
\(\Delta H\degree=166280Jmol^{-1}=166.28kJ\ mol{-1}\)
We can also use the graph to find the temperature (T) at which the pressure is 10^7. The temperature is 10^3 K.
We can substitute the values of ΔH° and T into equation (iii) to solve for ΔS°.
\(ln(\frac{P}{1}) = \frac{-\Delta H\degree}{RT} + \frac{\Delta S\degree}{R}\)
\(-3 = -\frac{2\times10^4\times R}{R\times 10^3} + \frac{\Delta S\degree}{R}\)
\(\frac{\Delta S\degree}{R}=20-3=17\)
\(\frac{\Delta S\degree}{R}=17\times R=17\times8.314=141.34JK^{-1}\ mol^{-1}\)
So, the correct answer is \(141.34JK^{-1}\ mol^{-1}\)
Top Questions on Law Of Chemical Equilibrium And Equilibrium Constant
For the reaction A(g) $\rightleftharpoons$ 2B(g), the backward reaction rate constant is higher than the forward reaction rate constant by a factor of 2500, at 1000 K.
[Given: R = 0.0831 atm $mol^{–1} K^{–1}$]
$K_p$ for the reaction at 1000 K is:- NEET (UG) - 2025
- Chemistry
- Law Of Chemical Equilibrium And Equilibrium Constant
- Higher yield of NO in the reaction
N$_2$ (g) + O$_2$ (g) $\rightarrow$ 2NO (g)
can be obtained at [$\Delta H$ of the reaction = +180.7 kJ mol$^{-1}$]- NEET (UG) - 2025
- Chemistry
- Law Of Chemical Equilibrium And Equilibrium Constant
- For the reaction $ N_2 + 3H_2 \rightleftharpoons 2NH_3 $, if initially 1 mole of $ N_2 $ and 3 moles of $ H_2 $ are taken and at equilibrium 0.4 moles of $ NH_3 $ are formed, find the equilibrium concentration of $ H_2 $.
- BITSAT - 2025
- Chemistry
- Law Of Chemical Equilibrium And Equilibrium Constant
- For the reaction \( \mathrm{N_2} + 3\mathrm{H_2} \rightleftharpoons 2\mathrm{NH_3} \), the equilibrium constant \(K_c\) is 0.5 at a certain temperature. If initially, 1 mole of \(\mathrm{N_2}\) and 3 moles of \(\mathrm{H_2}\) are placed in a 1 L container, what is the equilibrium concentration of \(\mathrm{NH_3}\)?
- BITSAT - 2025
- Chemistry
- Law Of Chemical Equilibrium And Equilibrium Constant
The equilibrium constant for decomposition of $ H_2O $ (g) $ H_2O(g) \rightleftharpoons H_2(g) + \frac{1}{2} O_2(g) \quad (\Delta G^\circ = 92.34 \, \text{kJ mol}^{-1}) $ is $ 8.0 \times 10^{-3} $ at 2300 K and total pressure at equilibrium is 1 bar. Under this condition, the degree of dissociation ($ \alpha $) of water is _____ $\times 10^{-2}$ (nearest integer value). [Assume $ \alpha $ is negligible with respect to 1]
- JEE Main - 2025
- Chemistry
- Law Of Chemical Equilibrium And Equilibrium Constant
Questions Asked in JEE Advanced exam
- If $$ \alpha = \int_{\frac{1}{2}}^{2} \frac{\tan^{-1} x}{2x^2 - 3x + 2} \, dx, $$ then the value of $ \sqrt{7} \tan \left( \frac{2\alpha \sqrt{7}}{\pi} \right) $ is.
(Here, the inverse trigonometric function $ \tan^{-1} x $ assumes values in $ \left( -\frac{\pi}{2}, \frac{\pi}{2} \right) $.)- JEE Advanced - 2025
- Integral Calculus
- Consider the vectors $$ \vec{x} = \hat{i} + 2\hat{j} + 3\hat{k},\quad \vec{y} = 2\hat{i} + 3\hat{j} + \hat{k},\quad \vec{z} = 3\hat{i} + \hat{j} + 2\hat{k}. $$ For two distinct positive real numbers $ \alpha $ and $ \beta $, define $$ \vec{X} = \alpha \vec{x} + \beta \vec{y} - \vec{z},\quad \vec{Y} = \alpha \vec{y} + \beta \vec{z} - \vec{x},\quad \vec{Z} = \alpha \vec{z} + \beta \vec{x} - \vec{y}. $$ If the vectors $ \vec{X}, \vec{Y}, \vec{Z} $ lie in a plane, then the value of $ \alpha + \beta - 3 $ is ________.
Let $ a_0, a_1, ..., a_{23} $ be real numbers such that $$ \left(1 + \frac{2}{5}x \right)^{23} = \sum_{i=0}^{23} a_i x^i $$ for every real number $ x $. Let $ a_r $ be the largest among the numbers $ a_j $ for $ 0 \leq j \leq 23 $. Then the value of $ r $ is ________.
- JEE Advanced - 2025
- binomial expansion formula
- The total number of real solutions of the equation $$ \theta = \tan^{-1}(2 \tan \theta) - \frac{1}{2} \sin^{-1} \left( \frac{6 \tan \theta}{9 + \tan^2 \theta} \right) $$ is
(Here, the inverse trigonometric functions $ \sin^{-1} x $ and $ \tan^{-1} x $ assume values in $[-\frac{\pi}{2}, \frac{\pi}{2}]$ and $(-\frac{\pi}{2}, \frac{\pi}{2})$, respectively.)- JEE Advanced - 2025
- Inverse Trigonometric Functions
A temperature difference can generate e.m.f. in some materials. Let $ S $ be the e.m.f. produced per unit temperature difference between the ends of a wire, $ \sigma $ the electrical conductivity and $ \kappa $ the thermal conductivity of the material of the wire. Taking $ M, L, T, I $ and $ K $ as dimensions of mass, length, time, current and temperature, respectively, the dimensional formula of the quantity $ Z = \frac{S^2 \sigma}{\kappa} $ is:
- JEE Advanced - 2025
- Dimensional Analysis
Concepts Used:
Law of Chemical Equilibrium
Law of Chemical Equilibrium states that at a constant temperature, the rate of a chemical reaction is directly proportional to the product of the molar concentrations of the reactants each raised to a power equal to the corresponding stoichiometric coefficients as represented by the balanced chemical equation.
Let us consider a general reversible reaction;
A+B ↔ C+D
After some time, there is a reduction in reactants A and B and an accumulation of the products C and D. As a result, the rate of the forward reaction decreases and that of backward reaction increases.
Eventually, the two reactions occur at the same rate and a state of equilibrium is attained.
By applying the Law of Mass Action;
The rate of forward reaction;
Rf = Kf [A]a [B]b
The rate of backward reaction;
Rb = Kb [C]c [D]d
Where,
[A], [B], [C] and [D] are the concentrations of A, B, C and D at equilibrium respectively.
a, b, c, and d are the stoichiometric coefficients of A, B, C and D respectively.
Kf and Kb are the rate constants of forward and backward reactions.
However, at equilibrium,
Rate of forward reaction = Rate of backward reaction.
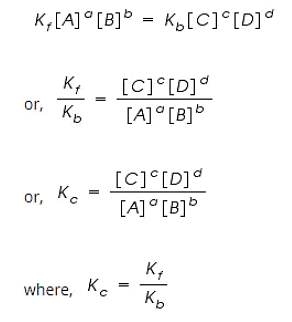
Kc is called the equilibrium constant expressed in terms of molar concentrations.
The above equation is known as the equation of Law of Chemical Equilibrium.