Question:
Find the area of a rhombus whose side is 6 cm and whose altitude is 4 cm. If one of its diagonals is 8 cm long, find the length of the other diagonal.
Find the area of a rhombus whose side is 6 cm and whose altitude is 4 cm. If one of its diagonals is 8 cm long, find the length of the other diagonal.
Updated On: Nov 30, 2023
Hide Solution
Verified By Collegedunia
Solution and Explanation
Let the length of the other diagonal of the rhombus be \(x\).
A rhombus is a special case of a parallelogram.
The area of a parallelogram is given by the product of its base and height.
Thus, area of the given rhombus = Base × Height
= 6 cm × 4 cm
= 24 cm2
Also, area of rhombus = \(\frac{1}{2} \) (Product of its diagonals)
24 = \(\frac{1}{2} (x × 8\) cm\()\)
\(x × 4 = 24\)
\(x = 6\) cm
Thus, the length of the other diagonal is 6 cm.
Was this answer helpful?
0
0
Top Questions on Area of a Polygon
- Top surface of a raised platform is in the shape of a regular octagon as shown in the figure. Find the area of the octagonal surface.

- CBSE Class VIII
- Mathematics
- Area of a Polygon
- The area of a trapezium is 34 cm2 and the length of one of the parallel sides is 10 cm and its height is 4 cm. Find the length of the other parallel side.

- CBSE Class VIII
- Mathematics
- Area of a Polygon
- The diagonals of a rhombus are 7.5 cm and 12 cm. Find its area.
- CBSE Class VIII
- Mathematics
- Area of a Polygon
- Diagram of the adjacent picture frame has outer dimensions = 24 cm x 28 cm and inner dimensions 16 cm x 20 cm. Find the area of each section of the frame, if the width of each section is same.
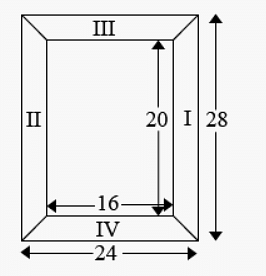
- CBSE Class VIII
- Mathematics
- Area of a Polygon
- There is a pentagonal shaped park as shown in the figure.
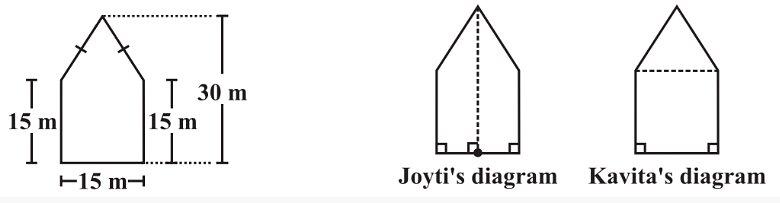
For finding its area Jyoti and Kavita divided it in two different ways. Find the area of this park using both ways. Can you suggest some other way of finding its area?- CBSE Class VIII
- Mathematics
- Area of a Polygon
View More Questions
Questions Asked in CBSE Class VIII exam
- Identify the part which vibrates to produce sound in the following instruments.
- Dholak
- Sitar
- Flute
- CBSE Class VIII
- Sound is Produced by a Vibrating Body
- Explain why objects moving in fluids must have special shapes.
- CBSE Class VIII
- Fluid Friction
- Find the number of digits in the square root of each of the following numbers (without any calculation).
- 64
- 144
- 4489
- 27225
- 390625
- CBSE Class VIII
- Square Roots of Decimals
- Find the least number which must be subtracted from each of the following numbers so as to get a perfect square. Also find the square root of the perfect square so obtained.
- 402
- 1989
- 3250
- 825
- 4000
- CBSE Class VIII
- Square Roots of Decimals
- Subtract 4p2q – 3pq + 5pq2 – 8p + 7q – 10 from 18 – 3p – 11q + 5pq – 2pq2 + 5p2q
- CBSE Class VIII
- Addition and Subtraction of Algebraic Expressions
View More Questions