There are four sellers namely Ram, Mohan, Lakhan and Raghav. Each of them has three types of articles (pen, pencil and erasers). The given table shows the sum of the cost prices (in Rs.) of each pen and pencil, difference between total cost prices (in Rs.) of all the pencils and erasers and sum of cost prices (in Rs.) of all the pens and erasers, with the respective sellers.
| Sellers | Sum of the cost price (in Rs.) of each pen and pencil | Cost price (in Rs.) of all pencils - Cost price (in Rs.) of all erasers | Sum of cost price (in Rs.) of all pens and erasers |
| Ram | 15 | 100 | 2100 |
| Mohan | 13 | 480 | 2050 |
| Lakhan | 27 | 600 | 3000 |
| Raghav | 19 | 300 | 3300 |
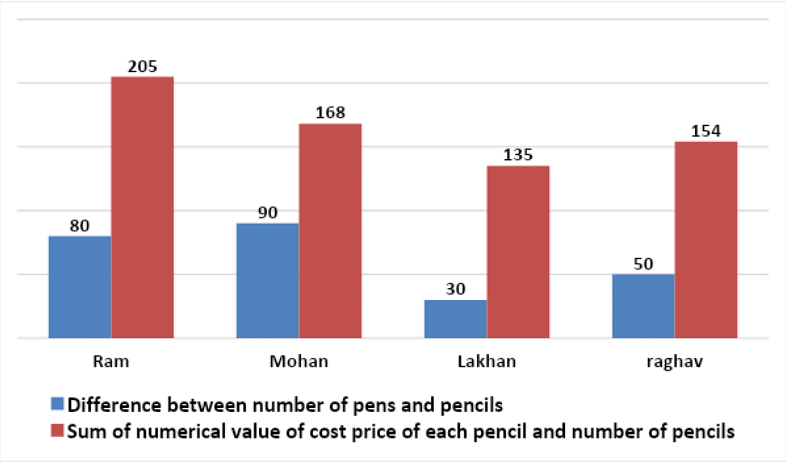
Note : Number of pens/pencils/erasers sold by each of the given sellers is an integer.
Out of total number of erasers with Ram, 180 remained unsold while he sold rest of the erasers at a profit of 25% each. If cost price of each eraser for Ram is Rs. 7 less than cost price of each pen for him, then find the selling price of all the erasers sold by him.
- Rs. 360
- Rs. 450
- Rs. 420
- Rs. 300
The Correct Option is B
Solution and Explanation
For Ram:
Let the number of pencils with him be ‘x’ and cost price of each pencil be Rs. ‘a’
Therefore, total cost price of all the pencils = Rs. ax
Total cost price of all the erasers = Rs. (ax – 100)
Total cost price of all the pens = 2100 – (ax – 100) = Rs. (2200 – ax)
Therefore, sum of cost price of all the pen and pencils = 2200 – ax + ax = Rs. 2200
Also, cost price of each pen = Rs. (15 – a)
Case 1: Let the number of pen be more than that of pencils
Therefore, number of pens = Rs. (x + 80)
According to the question,
(15 – a) × (x + 80) + ax = 2200
Or, 15x – 80a = 1000...... (1)
Or, x + a = 205...... (2)
On solving equation (1) and (2), we get
Cost price of each pencil = Rs. 2075/65 (not possible)
Case 2: Let the number of pen sold be less than number of pencils
Therefore, number of pens = (x – 80)
According to the question,
(x – 80) × (15 – a) + ax = 2200
Or, 15x + 80a = 3400.......... (3)
Also, x + a = 205...... (4)
On solving equation (3) and (4), we get
Cost price of each pencil = Rs. 5
Number of pencils = 200
Total cost price of all the pencils = 200 × 5 = Rs. 1000
Cost price of each pen = Rs. 10
Number pens = 120
Total cost price of all the pen = 120 × 10 = Rs. 1200
Total cost price of all the erasers = 1000 – 100 = Rs. 900
| Number of pens | Cost price (in Rs.) of each pen | Number of pencils | Cost price (in Rs.) of each pencil | Total cost price (in Rs.) of all erasers | |
| Ram | 120 | 10 | 200 | 5 | 900 |
| Mohan | 250 | 5 | 160 | 8 | 800 |
| Lakhan | 150 | 12 | 120 | 15 | 1200 |
| Raghav | 200 | 15 | 150 | 4 | 300 |
Cost price of each eraser = 10 – 7 = Rs. 3
Therefore, number of erasers with Ram = \(\frac{900}{3} \)= 300
Number of erasers sold = 300 – 180 = 120
Required selling price = 1.25 × 120 × 3 = Rs. 450.
If Mohan sold certain number of pencils which is equal to the difference between number of pencils with him and Ram, at 50% profit after giving a discount of 20%, then find the marked price of all the pencils sold by him.
- Rs. 600
- Rs. 480
- Rs. 300
- Rs. 800
The Correct Option is A
Solution and Explanation
The correct option is (A) : Rs. 600.
For Ram:
Let the number of pencils with him be ‘x’ and cost price of each pencil be Rs. ‘a’
Therefore, total cost price of all the pencils = Rs. ax
Total cost price of all the erasers = Rs. (ax – 100)
Total cost price of all the pens = 2100 – (ax – 100) = Rs. (2200 – ax)
Therefore, sum of cost price of all the pen and pencils = 2200 – ax + ax = Rs. 2200
Also, cost price of each pen = Rs. (15 – a)
Case 1: Let the number of pen be more than that of pencils
Therefore, number of pens = Rs. (x + 80)
According to the question,
(15 – a) × (x + 80) + ax = 2200
Or, 15x – 80a = 1000...... (1)
Or, x + a = 205...... (2)
On solving equation (1) and (2), we get
Cost price of each pencil = Rs. \(\frac{2075}{65}\) (not possible)
Case 2: Let the number of pen sold be less than number of pencils
Therefore, number of pens = (x – 80)
According to the question,
(x – 80) × (15 – a) + ax = 2200
Or, 15x + 80a = 3400.......... (3)
Also, x + a = 205...... (4)
On solving equation (3) and (4), we get
Cost price of each pencil = Rs. 5
Number of pencils = 200
Total cost price of all the pencils = 200 × 5 = Rs. 1000
Cost price of each pen = Rs. 10
Number pens = 120
Total cost price of all the pen = 120 × 10 = Rs. 1200
Total cost price of all the erasers = 1000 – 100 = Rs. 900
| Number of pens | Cost price (in Rs.) of each pen | Number of pencils | Cost price (in Rs.) of each pencil | Total cost price (in Rs.) of all erasers | |
| Ram | 120 | 10 | 200 | 5 | 900 |
| Mohan | 250 | 5 | 160 | 8 | 800 |
| Lakhan | 150 | 12 | 120 | 15 | 1200 |
| Raghav | 200 | 15 | 150 | 4 | 300 |
Number of pencils sold by Mohan = 200 – 160 = 40
Selling price of 40 pencils = 1.5 × 8 × 40 = Rs. 480
Marked price of 40 pencils =\(\frac{480}{0.8}\)= Rs. 600.
If the cost price of each pen for Lakhan had been Rs. 2 less, then the cost price of each eraser with him would have been 85% less than that of each pen with him. Find the sum of selling price of all the pencils and 120 erasers with Lakhan such that he sold each pencil at 20% profit and each eraser at profit of Rs. 2.5.
- Rs. 2140
- Rs. 4080
- Rs. 2320
- Rs. 2640
The Correct Option is D
Solution and Explanation
The correct option is (D) : Rs. 2640.
For Ram:
Let the number of pencils with him be ‘x’ and cost price of each pencil be Rs. ‘a’
Therefore, total cost price of all the pencils = Rs. ax
Total cost price of all the erasers = Rs. (ax – 100)
Total cost price of all the pens = 2100 – (ax – 100) = Rs. (2200 – ax)
Therefore, sum of cost price of all the pen and pencils = 2200 – ax + ax = Rs. 2200
Also, cost price of each pen = Rs. (15 – a)
Case 1: Let the number of pen be more than that of pencils
Therefore, number of pens = Rs. (x + 80)
According to the question,
(15 – a) × (x + 80) + ax = 2200
Or, 15x – 80a = 1000...... (1)
Or, x + a = 205...... (2)
On solving equation (1) and (2), we get
Cost price of each pencil = Rs. \(\frac{2075}{65}\) (not possible)
Case 2: Let the number of pen sold be less than number of pencils
Therefore, number of pens = (x – 80)
According to the question,
(x – 80) × (15 – a) + ax = 2200
Or, 15x + 80a = 3400.......... (3)
Also, x + a = 205...... (4)
On solving equation (3) and (4), we get
Cost price of each pencil = Rs. 5
Number of pencils = 200
Total cost price of all the pencils = 200 × 5 = Rs. 1000
Cost price of each pen = Rs. 10
Number pens = 120
Total cost price of all the pen = 120 × 10 = Rs. 1200
Total cost price of all the erasers = 1000 – 100 = Rs. 900
| Number of pens | Cost price (in Rs.) of each pen | Number of pencils | Cost price (in Rs.) of each pencil | Total cost price (in Rs.) of all erasers | |
| Ram | 120 | 10 | 200 | 5 | 900 |
| Mohan | 250 | 5 | 160 | 8 | 800 |
| Lakhan | 150 | 12 | 120 | 15 | 1200 |
| Raghav | 200 | 15 | 150 | 4 | 300 |
According to the question,
Cost price of each eraser with Lakhan = 0.85 × (12 – 2) = Rs. 1.5
Required sum = 1.2 × 15 ×120 + (1.5 + 2.5) × 120 = 2160 + 480 = Rs. 2640
Find the average number of erasers with Ram, Mohan and Lakhan such that cost price of each eraser with Ram is Rs. 3 which is Re. 1 more than cost price of each eraser with Mohan and twice the cost price of each eraser with Lakhan.
- 480
- 400
- 500
- 600
The Correct Option is C
Solution and Explanation
The correct option is (C) : 500
For Ram:
Let the number of pencils with him be ‘x’ and cost price of each pencil be Rs. ‘a’
Therefore, total cost price of all the pencils = Rs. ax
Total cost price of all the erasers = Rs. (ax – 100)
Total cost price of all the pens = 2100 – (ax – 100) = Rs. (2200 – ax)
Therefore, sum of cost price of all the pen and pencils = 2200 – ax + ax = Rs. 2200
Also, cost price of each pen = Rs. (15 – a)
Case 1: Let the number of pen be more than that of pencils
Therefore, number of pens = Rs. (x + 80)
According to the question,
(15 – a) × (x + 80) + ax = 2200
Or, 15x – 80a = 1000...... (1)
Or, x + a = 205...... (2)
On solving equation (1) and (2), we get
Cost price of each pencil = Rs. \(\frac{2075}{65}\) (not possible)
Case 2: Let the number of pen sold be less than number of pencils
Therefore, number of pens = (x – 80)
According to the question,
(x – 80) × (15 – a) + ax = 2200
Or, 15x + 80a = 3400.......... (3)
Also, x + a = 205...... (4)
On solving equation (3) and (4), we get
Cost price of each pencil = Rs. 5
Number of pencils = 200
Total cost price of all the pencils = 200 × 5 = Rs. 1000
Cost price of each pen = Rs. 10
Number pens = 120
Total cost price of all the pen = 120 × 10 = Rs. 1200
Total cost price of all the erasers = 1000 – 100 = Rs. 900
| Number of pens | Cost price (in Rs.) of each pen | Number of pencils | Cost price (in Rs.) of each pencil | Total cost price (in Rs.) of all erasers | |
| Ram | 120 | 10 | 200 | 5 | 900 |
| Mohan | 250 | 5 | 160 | 8 | 800 |
| Lakhan | 150 | 12 | 120 | 15 | 1200 |
| Raghav | 200 | 15 | 150 | 4 | 300 |
According to the question,
Number of erasers with Ram = \(\frac{900}{3}\)= 300
Number of erasers with Mohan = {\(\frac{800}{(3 – 1)}\)} = 400
Number of erasers with Lakhan = {\(\frac{(1200 × 2)}{3}\)} = 800
Required average = {\(\frac{(300 + 400 + 800)}{3}\)} = 500.
Find the average number of pencils with Ram, Mohan ad Raghav together.
- 130
- 170
- 150
- 190
The Correct Option is B
Solution and Explanation
For Ram:
Let the number of pencils with him be ‘x’ and cost price of each pencil be Rs. ‘a’
Therefore, total cost price of all the pencils = Rs. ax
Total cost price of all the erasers = Rs. (ax – 100)
Total cost price of all the pens = 2100 – (ax – 100) = Rs. (2200 – ax)
Therefore, sum of cost price of all the pen and pencils = 2200 – ax + ax = Rs. 2200
Also, cost price of each pen = Rs. (15 – a)
Case 1: Let the number of pen be more than that of pencils
Therefore, number of pens = Rs. (x + 80)
According to the question,
(15 – a) × (x + 80) + ax = 2200
Or, 15x – 80a = 1000...... (1)
Or, x + a = 205...... (2)
On solving equation (1) and (2), we get
Cost price of each pencil = Rs. \(\frac{2075}{65}\) (not possible)
Case 2: Let the number of pen sold be less than number of pencils
Therefore, number of pens = (x – 80)
According to the question,
(x – 80) × (15 – a) + ax = 2200
Or, 15x + 80a = 3400.......... (3)
Also, x + a = 205...... (4)
On solving equation (3) and (4), we get
Cost price of each pencil = Rs. 5
Number of pencils = 200
Total cost price of all the pencils = 200 × 5 = Rs. 1000
Cost price of each pen = Rs. 10
Number pens = 120
Total cost price of all the pen = 120 × 10 = Rs. 1200
Total cost price of all the erasers = 1000 – 100 = Rs. 900
| Number of pens | Cost price (in Rs.) of each pen | Number of pencils | Cost price (in Rs.) of each pencil | Total cost price (in Rs.) of all erasers | |
| Ram | 120 | 10 | 200 | 5 | 900 |
| Mohan | 250 | 5 | 160 | 8 | 800 |
| Lakhan | 150 | 12 | 120 | 15 | 1200 |
| Raghav | 200 | 15 | 150 | 4 | 300 |
Desired average = \(\frac{(200 + 160 + 150)}{3}\) = 170.
Top Questions on Data Interpretation
The above is a schematic diagram of walkways (indicated by all the straight-lines) and lakes (3 of them, each in the shape of rectangles – shaded in the diagram) of a gated area. Different points on the walkway are indicated by letters (A through P) with distances being OP = 150 m, ON = MN = 300 m, ML = 400 m, EL = 200 m, DE = 400 m.
The following additional information about the facilities in the area is known.
1. The only entry/exit point is at C.
2. There are many residences within the gated area; all of them are located on the path AH and ML with four of them being at A, H, M, and L.
3. The post office is located at P and the bank is located at B.- CAT - 2024
- Data Interpretation & Logical Reasoning (DILR)
- Data Interpretation
- Two students, Amiya and Ramya are the only candidates in an election for the position of class representative. Students will vote based on the intensity level of Amiya’s and Ramya’s campaigns and the type of campaigns they run. Each campaign is said to have a level of 1 if it is a staid campaign and a level of 2 if it is a vigorous campaign. Campaigns can be of two types, they can either focus on issues, or on attacking the other candidate.
If Amiya and Ramya both run campaigns focusing on issues, then
• The percentage of students voting in the election will be 20 times the sum of the levels of campaigning of the two students. For example, if Amiya and Ramya both run vigorous campaigns, then $20 × (2+2)\%$, that is, $80\%$ of the students will vote in the election.
• Among voting students, the percentage of votes for each candidate will be proportional to the levels of their campaigns. For example, if Amiya runs a staid (i.e., level 1) campaign while Ramya runs a vigorous (i.e., level 2) campaign, then Amiya will receive \(\frac{1}{3}\) of the votes cast, and Ramya will receive the other \(\frac{2}{3}\).
The above-mentioned percentages change as follows if at least one of them runs a campaign attacking their opponent.
• If Amiya runs a campaign attacking Ramya and Ramya runs a campaign focusing on issues, then $10\%$ of the students who would have otherwise voted for Amiya will vote for Ramya, and another $10\%$ who would have otherwise voted for Amiya, will not vote at all.
• If Ramya runs a campaign attacking Amiya and Amiya runs a campaign focusing on issues, then $20\%$ of the students who would have otherwise voted for Ramya will vote for Amiya, and another $5\%$ who would have otherwise voted for Ramya, will not vote at all.
• If both run campaigns attacking each other, then $10\%$ of the students who would have otherwise voted for them had they run campaigns focusing on issues, will not vote at all.- CAT - 2024
- Data Interpretation & Logical Reasoning (DILR)
- Data Interpretation
- Read the Passage carefully and answer the following questions.
An air conditioner (AC) unit has three operating modes: Eco, Normal, and Turbo. The power consumption in each mode is as follows: Eco mode consumes 1000 watts, Normal mode consumes 1200 watts, and Turbo mode consumes 1500 watts. Yesterday, the AC was in operation from 2 PM to 10 PM. From 2 PM to 6 PM it ran in Eco mode. From 6 PM to 8 PM, it ran in Normal mode. From 8 PM to 10 PM it ran in Turbo mode.- CAT - 2024
- Data Interpretation & Logical Reasoning (DILR)
- Data Interpretation
- Read the Passage carefully and answer the following questions
A health food store specializing in dietary supplements and whole grains recorded the following sales figures (in kilograms) for various food categories last month: Millets (M) = 500 kg at a cost price of 40/kg and selling price of 50/kg; Protein supplements (P) = 300 kg at a cost price of 80/kg and selling price of 100/kg; Vitamin supplements (V) = 200 kg at a cost price of 60/kg and selling price of 80/kg; Carbohydrates (C) = 600 kg at a cost price of 80/kg and selling price of 100/kg; Fats (F) = 100 kg at a cost price of 120/kg and selling price of 150/kg. Additionally, they sold 50 kg of mixed nuts (N) at a cost price of 100/kg and a selling price of 130/kg- CAT - 2024
- Data Interpretation & Logical Reasoning (DILR)
- Data Interpretation
- In a class of 40 students, the ratio of boys to girls is 3:2. The average marks scored by boys is 42, and that by girls is 46. What is the average marks scored by the whole class?
- CUET (UG) - 2024
- General Aptitude
- Data Interpretation
Questions Asked in CAT exam
- The sum of all real values of $k$ for which $(\frac{1}{8})^k \times (\frac{1}{32768})^{\frac{4}{3}} = \frac{1}{8} \times (\frac{1}{32768})^{\frac{k}{3}}$ is
- CAT - 2024
- Logarithms
- Amal and Vimal together can complete a task in 150 days, while Vimal and Sunil together can complete the same task in 100 days. Amal starts working on the task and works for 75 days, then Vimal takes over and works for 135 days. Finally, Sunil takes over and completes the remaining task in 45 days. If Amal had started the task alone and worked on all days, Vimal had worked on every second day, and Sunil had worked on every third day, then the number of days required to complete the task would have been
- CAT - 2024
- Time and Work
- Gopi marks a price on a product in order to make 20% profit. Ravi gets 10% discount on this marked price, and thus saves Rs 15. Then, the profit, in rupees, made by Gopi by selling the product to Ravi, is
- CAT - 2024
- Profit and Loss
- Sam can complete a job in 20 days when working alone. Mohit is twice as fast as Sam and thrice as fast as Ayana in the same job. They undertake a job with an arrangement where Sam and Mohit work together on the first day, Sam and Ayana on the second day, Mohit and Ayana on the third day, and this three-day pattern is repeated till the work gets completed. Then, the fraction of total work done by Sam is
- CAT - 2024
- Time and Work
- The number of all positive integers up to 500 with non-repeating digits is
- CAT - 2024
- Number Systems