An object of size 7.0 cm is placed at 27 cm in front of a concave mirror of focal length 18 cm. At what distance from the mirror should a screen be placed, so that a sharp focused image can be obtained? Find the size and the nature of the image.
Solution and Explanation
Object distance, \(u = −27\ cm\)
Object height, \(h = 7\ cm\)
Focal length, \(f = −18\ cm\)
According to the mirror formula,
\(\frac 1v+\frac 1u=\frac1f\)
\(\frac1v=\frac 1f-\frac 1u\)
\(\frac 1v=-\frac {1}{18}+\frac {1}{27}\)
\(\frac 1v=-\frac {1}{54}\)
\(v=-54\ cm\)
The screen should be placed at a distance of \(54\ cm\) in front of the given mirror.
Magnfication, \(m=-\frac {\text {Image\ distance}}{\text {Object\ distance}}\)
\(m =-\frac {54}{27}\)
\(m=-2\)
The negative value of magnification indicates that the image formed is real.
Magnfication, \(m=\frac {\text{Height\ of\ the\ image}}{\text {Height\ of\ the\ Object}}\)
\(m=\frac {h'}{h}\)
\(h'=m\times h\)
\(h'=7 \times (-2)\)
\(h'=-14\ cm\)
The negative value of image height indicates that the image formed is inverted.
Top Questions on Spherical Mirrors
- A concave mirror produces an image of an object such that the distance between the object and image is 20 cm. If the magnification of the image is \( -3 \), then the magnitude of the radius of curvature of the mirror is:
- JEE Main - 2025
- Physics
- Spherical Mirrors
- (ii) An object at a distance of 16 cm from a spherical mirror forms a virtual image at a distance of 12 cm behind the mirror. Determine the magnification of the image and type of the mirror.
- UP Board X - 2025
- Science
- Spherical Mirrors
- Image of an object formed by a concave mirror is real and of the size of the object. The object is placed -
- UP Board X - 2025
- Science
- Spherical Mirrors
- With the help of a suitable ray diagram, derive the formula \( \frac{1}{v} + \frac{1}{u} = \frac{1}{f} \) for a concave mirror.
- UP Board XII - 2025
- Physics
- Spherical Mirrors
- The length of the image formed by a concave mirror:
- UP Board XII - 2025
- Physics
- Spherical Mirrors
Questions Asked in CBSE X exam
- Name the method by which Amoeba and Leishmania reproduce. Write a major difference in the way they divide to produce new individuals.
- CBSE Class X - 2025
- How Do Organisms Reproduce
आप दिव्य/दिव्या हैं। आप अपनी आवासीय समिति के कल्याण सचिव हैं। अपनी सोसाइटी में आयोजित होने वाले सात दिवसीय योग शिविर की जानकारी देते हुए एक सूचना लिखिए।
- CBSE Class X - 2025
- पत्र लेखन
- Find the zeroes of the polynomial: \[ q(x) = 8x^2 - 2x - 3 \] Hence, find a polynomial whose zeroes are 2 less than the zeroes of \(q(x)\)
- CBSE Class X - 2025
- Polynomials
- Prove that: \[ \frac{\cos \theta - 2 \cos^3 \theta}{\sin \theta - 2 \sin^3 \theta} + \cot \theta = 0 \]
- CBSE Class X - 2025
- Trigonometric Identities
- Imagine you are a Village Development Officer of a village. Explain the usefulness of formal sources of credit for the farmers.
- CBSE Class X - 2025
- Money and Credit
Concepts Used:
Spherical Mirrors
A spherical mirror is a mirror which has been cut out of a spherical surface.
There are two kinds of spherical mirrors:
- Convex Mirror
- Concave Mirror
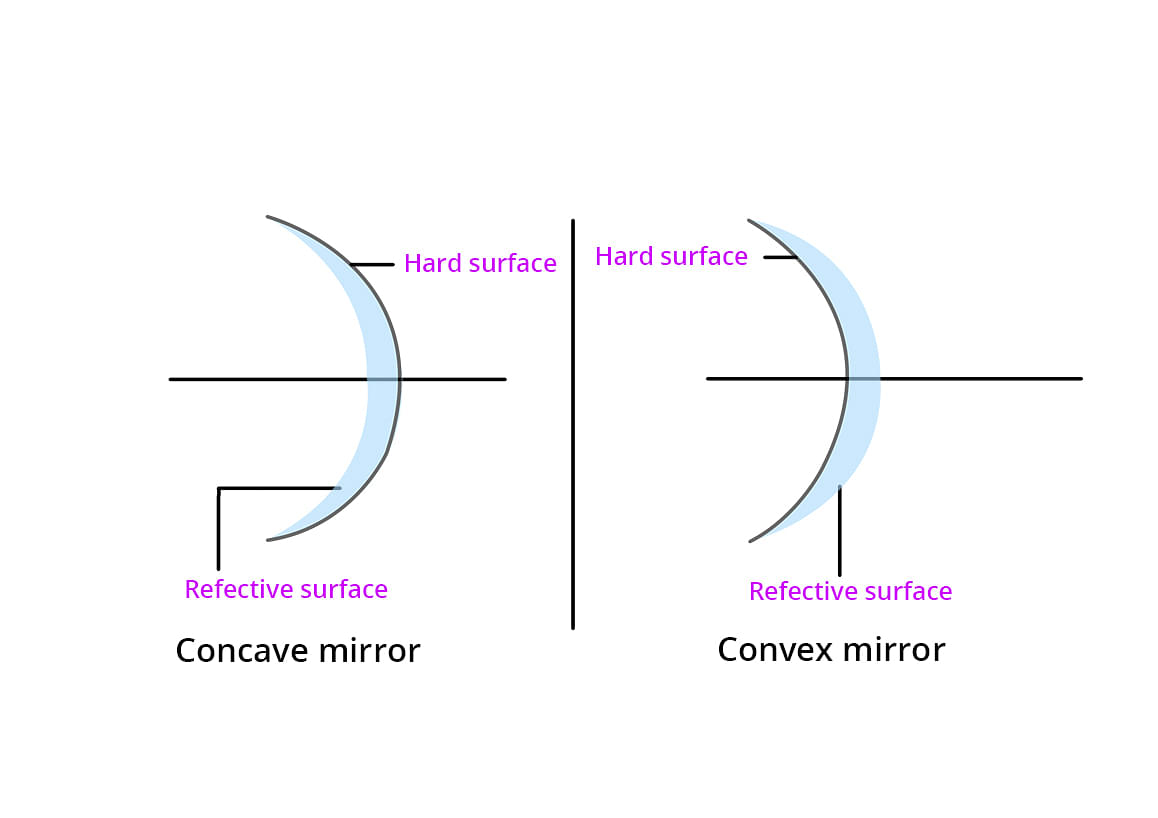
Concave Mirror
Concave mirrors are also called converging mirrors, because in these types of mirrors, light rays converge at a point after impact and reflect back from the reflective surface of the mirror.
Convex Mirror
The convex mirror has a reflective surface that is curved outward. Regardless of the distance between the subject and the mirrors, these mirrors are "always" virtual, upright and reduced.