Question

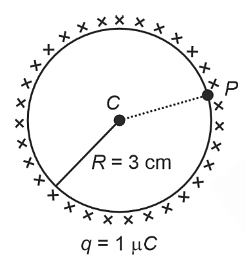
A thin spherical shell is charged by some source. The potential difference between the two points C and P (in V) shown in the figure is:
(Take \(\frac{1}{4}\pi\epsilon_0 = 9 × 109\)\(\frac{1}{4\pi\epsilon_0}=9\times10^9\) SI units)

• A. 3 × 10⁵
• B. 1 × 10⁵
• C. 0.5 × 10⁵
• D. Zero

Answer 1

The Correct Option is D

Charged Spherical Shell:

Inside, the electric field is zero, leading to constant potential.

Potential Formula: V = (1 / 4πε₀) * (q / R)

Given: q = 1 μC, R = 3 cm, 1/4πε₀ = 9 × 10⁹

Key Point: Potential inside and on the surface is equal.

Therefore: Potential difference (ΔV) between any inside point and surface point is zero.

Answer 2

Step 1: Recall the Property of a Spherical Shell 

Inside a charged spherical shell, the electric field is zero due to the symmetry of the charge distribution. Hence, the potential remains constant inside the shell.

Step 2: Potential Difference Between C and P

Since the electric field is zero inside the shell, the potential at any two points inside the shell, including C and P, is the same:

$$ V_C = V_P, \quad \Delta V = V_C - V_P = 0 $$

Step 3: Conclude

The potential difference between C and P is 0V.