Question

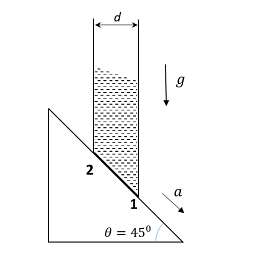
A cylindrical tube, with its base as shown in the figure, is filled with water It is moving down with a constant acceleration $a$ along a fixed inclined plane with angle $\theta=45^{\circ} P_{1}$ and $P_{2}$ are pressures at points $1$ and $2$, respectively located at the base of the tube. Let $\beta=\left(P_{1}-P_{2}\right) /(\rho g d)$, where $\rho$ is density of water, $d$ is the inner diameter of the tube and $g$ is the acceleration due to gravity. Which of the following statement(s) is(are) correct?

• A. $\beta=0$ when $a=\frac {g}{\sqrt{2}}$
• B. $\beta > 0$ when $a=\frac{g}{\sqrt{2}}$
• C. $\beta=\frac{\sqrt{2}-1}{\sqrt{2}}$ when $a =\frac{g}{2}$
• D. $\beta=\frac{1}{\sqrt{2}}$ when $a = \frac{g}{2}$

Answer 1

The Correct Option is A, C

The correct answer is option
(A): $\beta=0$ when $a=\frac {g}{\sqrt{2}}$
(C): $\beta=\frac{\sqrt{2}-1}{\sqrt{2}}$ when $a =\frac{g}{2}$