Two wires of diameter 0.25 cm, one made of steel and the other made of brass are loaded as shown in Fig. 8.11. The unloaded length of steel wire is 1.5 m and that of brass wire is 1.0 m. Compute the elongations of the steel and the brass wires.

Two wires of diameter 0.25 cm, one made of steel and the other made of brass are loaded as shown in Fig. 8.11. The unloaded length of steel wire is 1.5 m and that of brass wire is 1.0 m. Compute the elongations of the steel and the brass wires.

Solution and Explanation
Elongation of the steel wire = 1.49 × 10–4 m
Elongation of the brass wire = 1.3 × 10–4 m
Diameter of the wires, d = 0.25 m
Hence, the radius of the wires, r = \(\frac{d }{ 2}\) = 0.125 cm
Length of the steel wire, L1 = 1.5 m
Length of the brass wire, L2 = 1.0 m
Total force exerted on the steel wire:
F1 = (4 + 6) g = 10 × 9.8 = 98 N
Young’s modulus for steel :
\(Y1 =\frac{ (\frac{F_1 }{ A_1}) }{ (\frac{ΔL_1 }{ L_1})}\)
Where, ΔL1 = Change in the length of the steel wire
A1 = Area of cross-section of the steel wire = \(πr_1^2\)
Young’s modulus of steel, Y1 = 2.0 × 10 11 Pa
∴ ΔL1 =\(\frac{ F_1 × L_1 }{A_1 × Y_1} = \frac{F_1 × L_1 }{ πr_1^2 × Y_1 }\)
= \(\frac{98 × 1.5 }{ π(0.125 × 10 ^{- 2})^2 × 2 × 10 ^{11}}\) = 1.49 × 10 - 4 m
Total force on the brass wire : F2 = 6 × 9.8 = 58.8 N
Young’s modulus for brass :
\(Y2 =\frac{ (\frac{F2 }{ A2})}{(\frac{ΔL_2 }{ L_2})}\)
Where,
ΔL2 = Change in length
A2 = Area of Cross - section of the brass wire
∴ ΔL2 = \(\frac{F_2 × L_2 }{ A_2 × Y_2 }=\frac{ F_2 × L_2 }{ πr_2^2 × Y_2 }\)
= \(\frac{58.8 × 1.0 }{ π × (0.125 × 10 ^{- 2})^2 × (0.91 × 10^{ 11}) }\)= 1.3 × 10 - 4 m
Elongation of the steel wire = 1.49 × 10 - 4 m
Elongation of the brass wire = 1.3 × 10 - 4 m
Top Questions on elastic moduli
- The slope of the stress-strain curve in the elastic deformation region is
- JKCET - 2024
- Physics
- elastic moduli
- The law which states that within elastic limits strain produced is proportional to the stress producing it is known as
- JKCET - 2024
- Physics
- elastic moduli
- A wire of length L and radius r is clamped rigidly at one end. When the other end of the wire is pulled by a force F, its length increases by 5 cm. Another wire of the same material of length 4L and radius 4r is pulled by a force 4F under same conditions. The increase in length of this wire is ___ cm.
- JEE Main - 2022
- Physics
- elastic moduli
The elastic behavior of material for linear stress and linear strain, is shown in the figure. The energy density for a linear strain of 5×10–4 is ____ kJ/m3. Assume that material is elastic up to the linear strain of 5×10–4
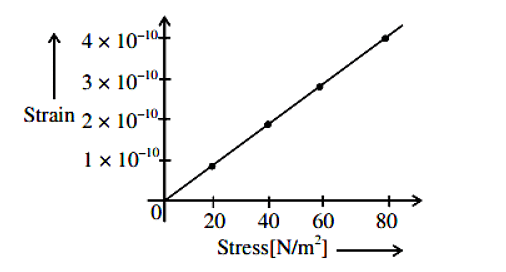
- JEE Main - 2022
- Physics
- elastic moduli
- If the length of a wire is made double and radius is halved of its respective values. Then, the Young’s modulus of the material of the wire will :
- JEE Main - 2022
- Physics
- elastic moduli
Questions Asked in CBSE Class XI exam
- Take one flower of the family Solanaceae and write its semi-technical description. Also draw their floral diagram.
- CBSE Class XI
- The Flower
- Write the resonance structures for SO3 , NO2 and NO3-
- CBSE Class XI
- Kossel-Lewis Approach to Chemical Bonding
- Have you known someone like the author’s grandmother? Do you feel the same sense of loss with regard to someone whom you have loved and lost?
- CBSE Class XI
- The Portrait of a lady
- The enthalpy of combustion of methane, graphite and dihydrogen at 298 K are, -890.3 kJ mol-1, -393.5 kJ mol-1, and -285.8 kJ mol-1 respectively. Enthalpy of formation of CH4(g) will be
- CBSE Class XI
- Thermodynamics terms
- (i) List the deeds that led Ray Johnson to describe Akhenaten as “wacky”.
(ii) What were the results of the CT scan?
(iii) List the advances in technology that have improved forensic analysis.
(iv) Explain the statement, “King Tut is one of the first mummies to be scanned — in death, as in life...”- CBSE Class XI
- Discovering Tut: The saga Continues