The formula of a metal oxide is M0.96 O1. The fractions of metal that exists as M3+and M2+ ions in that oxide are respectively
The formula of a metal oxide is M0.96 O1. The fractions of metal that exists as M3+and M2+ ions in that oxide are respectively
0.083, 0.916
0.916, 0.083
0.88,0.12
0.12, 0.88
The Correct Option is A
Solution and Explanation
The correct option is: (A): 0.083, 0.916.
The given metal oxide has the formula M0.96O1, indicating that there is a slight deficiency of the metal. This implies that the metal can exist in multiple oxidation states.
Since the oxide formula is M0.96O1, the ratio of metal ions to oxygen ions is not exactly 1:1. This suggests the presence of both M3+ and M2+ ions to balance the charges.
To justify the answer:
Fraction of M3+ ions: Since there is a slight deficiency of metal, indicating the presence of a higher oxidation state, the fraction of M3+ ions is lower. The difference between the ideal ratio of 1:1 and the actual composition indicates the fraction of M3+ ions: (1 - 0.96) = 0.04.
Fraction of M2+ ions: The remaining metal ions must be in the M2+ oxidation state. The difference between the ideal ratio and the M2+ fraction can be calculated as 1 - 0.04 = 0.96. So, the fractions of M3+ and M2+ ions are approximately 0.04 and 0.96
Top Questions on General Principles and Processes of Isolation of Elements
- The incorrect statement about the Hall-Heroult process is:
- KCET - 2024
- Chemistry
- General Principles and Processes of Isolation of Elements
- A particle of mass \( 2 \) kg is on a smooth horizontal table and moves in a circular path of radius \( 0.6 \) m. The height of the table from the ground is \( 0.8 \) m. If the angular speed of the particle is \( 12 \) rad/s, the magnitude of its angular momentum about a point on the ground right under the center of the circle is:
- BITSAT - 2024
- Physics
- General Principles and Processes of Isolation of Elements
A parallel plate capacitor with plate area \(A\) and plate separation \(d = 2\) m has a capacitance of \(4\mu F\). The new capacitance of the system if half of the space between them is filled with a dielectric material of dielectric constant \(K = 3\) (as shown in the figure) will be:
- BITSAT - 2024
- Physics
- General Principles and Processes of Isolation of Elements
- An oil drop of radius \(1 \, \mu{m}\) is held stationary under a constant electric field of \(3.65 \times 10^4 \, {N/C}\) due to some excess electrons present on it. If the density of the oil drop is \(1.26 \, {g/cm}^3\), then the number of excess electrons on the oil drop approximately is:
[Take, g = 10 m/s2]- BITSAT - 2024
- Physics
- General Principles and Processes of Isolation of Elements
A block of mass 1 kg is pushed up a surface inclined to horizontal at an angle of \( 60^\circ \) by a force of 10 N parallel to the inclined surface. When the block is pushed up by 10 m along the inclined surface, the work done against frictional force is:
[Given: \( g = 10 \) m/s\( ^2 \), \( \mu_s = 0.1 \)]
- BITSAT - 2024
- Physics
- General Principles and Processes of Isolation of Elements
Questions Asked in TS EAMCET exam
- The number of ways in which 15 identical gold coins can be distributed among 3 persons such that each one gets at least 3 gold coins is
- TS EAMCET - 2024
- permutations and combinations
- Identify the incorrect pair:
- TS EAMCET - 2024
- Plant Physiology
- The internal and external diameters of a hollow cylinder measured with vernier calipers are (5.73 \(\pm\) 0.01) cm and (6.01 \(\pm\) 0.01) cm respectively. Then the thickness of the cylinder wall is
- TS EAMCET - 2024
- Units and measurement
- f is a real valued function satisfying the relation \(f\bigl(3x + \tfrac{1}{2x}\bigr) \;=\; 9x^2 + \tfrac{1}{4x^2}\). If \(f\bigl(x + \tfrac{1}{x}\bigr) = 1\) then \(x =\) ?
- TS EAMCET - 2024
- Relations and functions
- A body is projected from the ground at an angle of \( \tan^{-1}\sqrt{7} \) with the horizontal. At half of the maximum height, the speed of the body is \( n \) times the speed of projection. The value of \( n \) is
- TS EAMCET - 2024
- laws of motion
Concepts Used:
General Principles and Processes of Isolation of Elements
What are Ores and Minerals?
Minerals are the naturally occurring, homogeneous inorganic solid substances. They are having a definite chemical composition and crystalline structure, hardness and color. For example, copper pyrite, calamine, etc.
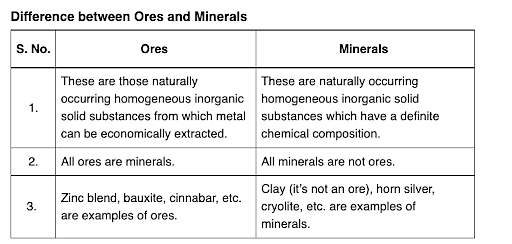
Impurities in an ore are called gauge. The removal of a gauge from the ore is called concentration ore.
Several steps are involved in the extraction of pure metal from ores. Major steps are as follows –
- Concentration of the ore
- Isolation of the metal from its concentrated ore
- Purification of the metal