In triangle ABC, ‘D’ and ‘E’ are two points on sides AB and AC, respectively such that DE is parallel to BC. If AD=16 cm, BD=(5x-16) cm, AE=2x cm and EC=(25-2x) cm, then find the value of ‘x’.
- \(2\sqrt10\)
- \(3\sqrt5\)
- \(4\sqrt5\)
- \(3\sqrt10\)
The Correct Option is A
Solution and Explanation
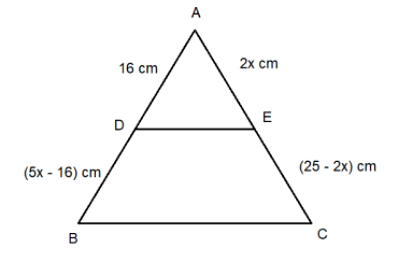
In triangle ABC, DE is parallel to BC
Therefore, triangle ADE is similar to triangle ABC
Therefore,
\((\frac{AD}{AB}) = (\frac{AE}{AC})\)
Or, {\(\frac{16}{(5x – 16 + 16)}\)} = {\(\frac{2x}{(25 – 2x + 2x)}\)}
Or, 5x × 2x = 16 × 25
Or, 10x2 = 400
Or, x2 = 40
Or, x = \(2\sqrt{10}\) (Since, length cannot be negative).
Top Questions on Geometry
Find the number of triangles in the given figure.

A regular dodecagon (12-sided regular polygon) is inscribed in a circle of radius \( r \) cm as shown in the figure. The side of the dodecagon is \( d \) cm. All the triangles (numbered 1 to 12 in the figure) are used to form squares of side \( r \) cm, and each numbered triangle is used only once to form a square. The number of squares that can be formed and the number of triangles required to form each square, respectively, are:

In the given figure, the numbers associated with the rectangle, triangle, and ellipse are 1, 2, and 3, respectively. Which one among the given options is the most appropriate combination of \( P \), \( Q \), and \( R \)?

- What is the area of a triangle with base \( 12 \, \text{cm} \) and height \( 8 \, \text{cm} \)?
- A solid trophy, consisting of two parts, has been designed in the following manner: the bottom part is a frustum of a cone with the bottom radius 30 cm, the top radius 20 cm, and height 40 cm, while the top part is a hemisphere with radius 20 cm. Moreover, the flat surface of the hemisphere is the same as the top surface of the frustum. If the entire trophy is to be gold-plated at the cost of Rs. 40 per square cm, what would the cost for gold-plating be closest to?
Questions Asked in CAT exam
- The sum of all real values of $k$ for which $(\frac{1}{8})^k \times (\frac{1}{32768})^{\frac{4}{3}} = \frac{1}{8} \times (\frac{1}{32768})^{\frac{k}{3}}$ is
- CAT - 2024
- Logarithms
- ABCD is a rectangle with sides AB = 56 cm and BC = 45 cm, and E is the midpoint of side CD. Then, the length, in cm, of radius of incircle of \(\triangle ADE\) is
- CAT - 2024
- Mensuration
- Let $x$, $y$, and $z$ be real numbers satisfying
\(4(x^2 + y^2 + z^2) = a,\)
\(4(x - y - z) = 3 + a.\)
Then $a$ equals ?- CAT - 2024
- Algebra
When $10^{100}$ is divided by 7, the remainder is ?
- CAT - 2024
- Basics of Numbers
- If $(a + b\sqrt{n})$ is the positive square root of $(29 - 12\sqrt{5})$, where $a$ and $b$ are integers, and $n$ is a natural number, then the maximum possible value of $(a + b + n)$ is ?
- CAT - 2024
- Algebra