Illustrate the law of conservation of energy by discussing the energy changes which occur when we draw a pendulum bob to one side and allow it to oscillate. Why does the bob eventually come to rest? What happens to its energy eventually? Is it a violation of the law of conservation of energy?
Solution and Explanation
In the case of pendulum energy in the Bob at any instant of time, can be either potential energy (P.E) or kinetic energy (K.E) or a mixture of both but its total energy at any instant of time remains constant. This can be illustrated by below illustration in the figure.
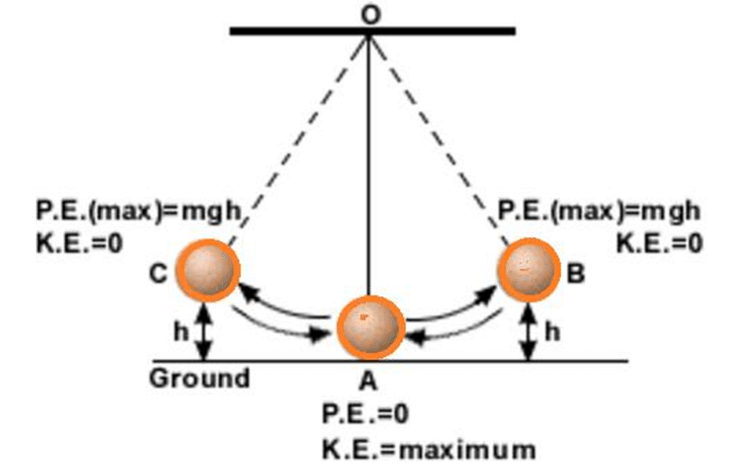
In case of pendulum energy in the Bob at any instant of time when the pendulum Bob is at position B.It has only potential energy(P.E) and no kinetic energy(K.E).
As the Bob starts moving from position B to position A potential energy(P.E) decreases but Kinetic Energy(K.E) increases.
When the Bob reaches position A, there is only kinetic energy(K.e) but no potential energy(P.E).
As Bob moves from position A to position C, potential energy(p.E) increases but kinetic energy decreases.
When Bob reaches position C, Bob stops for a very small instant of time, At that time, Bob has only potential energy but no kinetic energy.
The Bob eventually comes to rest due to the frictional force offered by the air and the rigid support holding the thread.
It is not a violation of the law of conservation of energy since mechanical energy can get converted into another form of energy which cannot be utilized for useful work. This loss of energy is called dissipation of energy.
Top Questions on Energy
- Which one of the following is widely used as fuel to produce nuclear energy?
- Match List-I with List-II:

- During the process of conversion from liquid to solid, the internal energy of the water
- Two sources of non-conventional energy
- A steel ball of mass 200 g falls freely from a height of 20 m and bounces to a height of 10.8 m from the ground. If the energy lost in this process is absorbed by the ball, the rise in its temperature is:
Questions Asked in CBSE Class IX exam
- Verify : (i) x 3 + y 3 = (x + y) (x 2 – xy + y 2 ) (ii) x 3 – y 3 = (x – y) (x 2 + xy + y 2 ).
- CBSE Class IX
- Algebraic Identities
- An object of mass 1 kg travelling in a straight line with a velocity of 10 m s–1 collides with, and sticks to, a stationary wooden block of mass 5 kg. Then they both move off together in the same straight line. Calculate the total momentum just before the impact and just after the impact. Also, calculate the velocity of the combined object.
- CBSE Class IX
- Inertia and Mass
- Discuss what these phrases mean to you.
(i) a yellow wood
(ii) it was grassy and wanted wear
(iii) the passing there
(iv) leaves no step had trodden black
(v) how way leads on to way- CBSE Class IX
- The fun they had
- What do you think Dinamani is the name of? Give a reason for your answer.
- CBSE Class IX
- My childhood
- (i) His thoughts when he looks into the mirror
(ii) His thoughts when the snake is coiled around his arm- CBSE Class IX
- The snake and the mirror
Concepts Used:
Work-Energy Theorem
The work and kinetic energy principle (also known as the work-energy theorem) asserts that the work done by all forces acting on a particle equals the change in the particle's kinetic energy. By defining the work of the torque and rotational kinetic energy, this definition can be extended to rigid bodies.
The change in kinetic energy KE is equal to the work W done by the net force on a particle is given by,
W = ΔKE = ½ mv2f − ½ mv2i
Where,
vi → Speeds of the particle before the application of force
vf → Speeds of the particle after the application of force
m → Particle’s mass
Note: Energy and Momentum are related by, E = p2 / 2m.