Question:

If the \(XZ\)-plane divides the straight line joining the points \((2,4,7)\) and \((3,-5,8)\) in the ratio \(α:1\),then the value of \(α\) is ?

Updated On: Apr 3, 2025

\(\dfrac{5}{4}\)
\(\dfrac{1}{3}\)
\(\dfrac{7}{8}\)
\(\dfrac{4}{5}\)
\(\dfrac{5}{2}\)

Hide Solution

Verified By Collegedunia

The Correct Option is D

Approach Solution - 1

Given that

Let the \(XZ \) plane divide the line segment joining points \((2,4,7) \) and \((3,−5,8)\) in the ratio \(∝:1.\)

Hence, by section formula, the coordinates of point of intersection are given by :

\((\dfrac{k(3)+2}{k+1},\dfrac{k(−5)+4}{k+1},\dfrac{k(8)+7}{k+1})\)

On the \(XZ \) plane, the \(y\)-coordinate of any point is zero.

,\(\dfrac{k(-5)+4}{k+1}=0\)

⇒ \(-5k+4=0\)

⇒ \(k=\dfrac{4}{5}\) (_Ans)

Was this answer helpful?

Hide Solution

Verified By Collegedunia

Approach Solution -2

We are given two points \( A(2, 4, 7) \) and \( B(3, -5, 8) \). The \( xz \)-plane divides the line segment joining these points in the ratio \( \alpha : 1 \).

The \( xz \)-plane is defined by the equation \( y = 0 \). The point \( P \) that divides the line segment \( AB \) in the ratio \( \alpha : 1 \) is given by:

\[ P \left( \frac{3\alpha + 2}{\alpha + 1}, \frac{-5\alpha + 4}{\alpha + 1}, \frac{8\alpha + 7}{\alpha + 1} \right) \]

Since \( P \) lies on the \( xz \)-plane, its \( y \)-coordinate must be zero:

\[ \frac{-5\alpha + 4}{\alpha + 1} = 0 \]

Solve for \( \alpha \):

\[ -5\alpha + 4 = 0 \]

\[ 5\alpha = 4 \]

\[ \alpha = \frac{4}{5} \]

The value of \( \alpha \) is \( \frac{4}{5} \).

The correct answer is:

(D) \( \frac{4}{5} \)

Was this answer helpful?

Questions Asked in KEAM exam

View More Questions

Concepts Used:

Straight lines

A straight line is a line having the shortest distance between two points.

A straight line can be represented as an equation in various forms, as show in the image below:

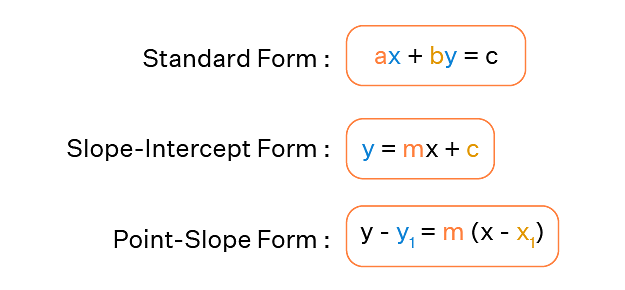
The following are the many forms of the equation of the line that are presented in straight line-

1. Slope – Point Form

Assume P0(x0, y0) is a fixed point on a non-vertical line L with m as its slope. If P (x, y) is an arbitrary point on L, then the point (x, y) lies on the line with slope m through the fixed point (x0, y0) if and only if its coordinates fulfil the equation below.

y – y₀ = m (x – x₀)

2. Two – Point Form

Let's look at the line. L crosses between two places. P₁(x₁, y₁) and P₂(x₂, y₂) are general points on L, while P (x, y) is a general point on L. As a result, the three points P₁, P₂, and P are collinear, and it becomes

The slope of P₂P = The slope of P₁P₂, i.e.

\(\frac{y-y_1}{x-x_1} = \frac{y_2-y_1}{x_2-x_1}\)

Hence, the equation becomes:

y - y₁ =\( \frac{y_2-y_1}{x_2-x_1} (x-x1)\)

3. Slope-Intercept Form

Assume that a line L with slope m intersects the y-axis at a distance c from the origin, and that the distance c is referred to as the line L's y-intercept. As a result, the coordinates of the spot on the y-axis where the line intersects are (0, c). As a result, the slope of the line L is m, and it passes through a fixed point (0, c). The equation of the line L thus obtained from the slope – point form is given by

y – c =m( x - 0 )

As a result, the point (x, y) on the line with slope m and y-intercept c lies on the line, if and only if

y = m x +c