If ax + by + c = 0 is normal to xy = 1, then determine if a and b are less than, greater than, or equal to zero.
If ax + by + c = 0 is normal to xy = 1, then determine if a and b are less than, greater than, or equal to zero.
Solution and Explanation
Step 1: Find the slope of the line \( ax + by + c = 0 \):
The given line is \( ax + by + c = 0 \). We can rewrite it in slope-intercept form \( y = mx + b \) by solving for \( y \):
\[
y = \frac{-a}{b}x - \frac{c}{b}
\]
Therefore, the slope of the line is \( m = -\frac{a}{b} \).
Step 2: Find the slope of the curve \( xy = 1 \):
The given curve is \( xy = 1 \), which can be rewritten as:
\[
y = \frac{1}{x}
\]
This is a hyperbola, and its slope at any point \( (x, y) \) is given by the derivative of \( y = x^{-1} \). Differentiating \( y = x^{-1} \), we get:
\[
\frac{dy}{dx} = -x^{-2} = -\frac{1}{x^2}
\]
Therefore, the slope of the curve at any point on the curve is \( -\frac{1}{x^2} \).
However, for the purposes of this problem, we are concerned with the condition that the line is normal to the curve at some point. A line normal to the curve has a slope that is the negative reciprocal of the slope of the curve.
Step 3: Condition for normality:
For the line \( ax + by + c = 0 \) to be normal to the curve \( xy = 1 \), the product of the slopes of the line and the curve at the point of intersection must be equal to \( -1 \).
The slope of the line is \( -\frac{a}{b} \), and the slope of the curve is \( -\frac{1}{x^2} \). For the line to be normal to the curve, we require:
\[
\left( -\frac{a}{b} \right) \times \left( -\frac{1}{x^2} \right) = -1
\]
Simplifying:
\[
\frac{a}{b} \cdot \frac{1}{x^2} = 1
\]
Step 4: Solve for the relationship between \( a \) and \( b \):
Since \( x^2 \) cannot be zero, the above equation implies that:
\[
\frac{a}{b} = 1
\]
Therefore, we conclude that \( a = b \), or equivalently, \( a \) and \( b \) must be of the same sign (either both positive or both negative).
Conclusion:
The relationship between \( a \) and \( b \) is that \( a \) and \( b \) must either both be positive or both be negative for the line \( ax + by + c = 0 \) to be normal to the curve \( xy = 1 \).
Top Questions on Straight lines
- If the slope of one of the pair of lines represented by \( 2x^2 + 3xy + Ky^2 = 0 \) is 2, then the angle between the pair of lines is:
- AP EAMCET - 2024
- Mathematics
- Straight lines
- The length of x-intercept made by the pair of lines \( 2x^2 + xy - 6y^2 - 2x + 17y - 12 = 0 \) is:
- AP EAMCET - 2024
- Mathematics
- Straight lines
- Two straight lines \( 3x - 2y = 5 \) and \( 2x + ky + 7 = 0 \) are perpendicular to each other. The value of \( k \) is:
- JEECUP - 2024
- Mathematics
- Straight lines
- If (1,5) is the midpoint of the segment of a line between the lines 5x −y −4 = 0 and 3x +4y −4=0,then the equation of the line will be:
- WBJEE - 2024
- Mathematics
- Straight lines
- The perpendicular distance between two parallel lines \( 3x + 4y - 6 = 0 \) and \( 6x + 8y + 7 = 0 \) is equal to:
- JEECUP - 2024
- Mathematics
- Straight lines
Questions Asked in MHT CET exam
- If $ f(x) = 2x^2 - 3x + 5 $, find $ f(3) $.
- MHT CET - 2025
- Functions
- Total pressure of the solution is 500, the partial pressure of component A is 400, and the partial pressure of component B is 575. What is the mole fraction of component B?
- MHT CET - 2025
- Mole concept and Molar Masses
- A block of mass \( 5 \, \text{kg} \) is placed on a horizontal surface. The coefficient of friction between the block and the surface is \( 0.4 \). What is the force of friction acting on the block?
- MHT CET - 2025
- Friction
- If $ \mathbf{a} $ and $ \mathbf{b} $ are non-coplanar unit vectors such that $ \mathbf{a} \times (\mathbf{b} \times \mathbf{c}) = \frac{\mathbf{b}}{2} $ then the angle between $ \mathbf{a} $ and $ \mathbf{b} $ is:
- MHT CET - 2025
- Vector Algebra
- A 5 kg block is placed on a horizontal surface. A force of 10 N is applied to the block. The coefficient of friction between the block and the surface is 0.2. Find the acceleration of the block.
- MHT CET - 2025
- laws of motion
Concepts Used:
Straight lines
A straight line is a line having the shortest distance between two points.
A straight line can be represented as an equation in various forms, as show in the image below:
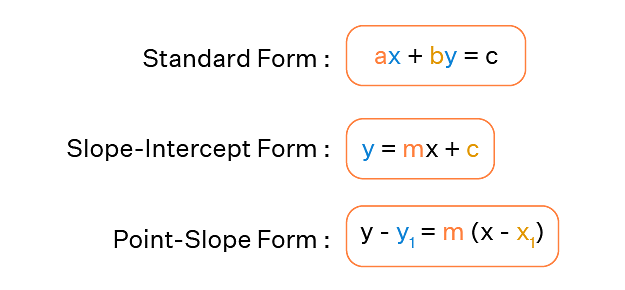
The following are the many forms of the equation of the line that are presented in straight line-
1. Slope – Point Form
Assume P0(x0, y0) is a fixed point on a non-vertical line L with m as its slope. If P (x, y) is an arbitrary point on L, then the point (x, y) lies on the line with slope m through the fixed point (x0, y0) if and only if its coordinates fulfil the equation below.
y – y0 = m (x – x0)
2. Two – Point Form
Let's look at the line. L crosses between two places. P1(x1, y1) and P2(x2, y2) are general points on L, while P (x, y) is a general point on L. As a result, the three points P1, P2, and P are collinear, and it becomes
The slope of P2P = The slope of P1P2 , i.e.
\(\frac{y-y_1}{x-x_1} = \frac{y_2-y_1}{x_2-x_1}\)
Hence, the equation becomes:
y - y1 =\( \frac{y_2-y_1}{x_2-x_1} (x-x1)\)
3. Slope-Intercept Form
Assume that a line L with slope m intersects the y-axis at a distance c from the origin, and that the distance c is referred to as the line L's y-intercept. As a result, the coordinates of the spot on the y-axis where the line intersects are (0, c). As a result, the slope of the line L is m, and it passes through a fixed point (0, c). The equation of the line L thus obtained from the slope – point form is given by
y – c =m( x - 0 )
As a result, the point (x, y) on the line with slope m and y-intercept c lies on the line, if and only if
y = m x +c