Draw a quadrilateral in the Cartesian plane, whose vertices are (-4, 5), (0, 7), (5, -5) and (-4, -2). Also, find its area.
Solution and Explanation
Let ABCD be the given quadrilateral with vertices A (-4, 5), B (0, 7), C (5,-5), and D (- 4, - 2).
Then, by plotting A, B, C, and D on the Cartesian plane and joining AB, BC, CD, and DA, the given quadrilateral can
be drawn as

To find the area of quadrilateral ABCD, we draw one diagonal, say AC.
Accordingly, area (ABCD) = area (ΔABC) + area (ΔACD)
We know that the area of a triangle whose vertices are (x1, y1), (x2, y2), and (x3, y3) is
\(\frac{1}{2}|x_1(y_2-y_3)+x_2(y_3-y_1)+x_3(y_1-y_2)|\)
Therefore, area of ΔABC
\(=\frac{1}{2}|-4(7+5)+0(-5-5)+5(5-7)|\, unit^2\)
\(=\frac{1}{2}|-4(12)+5(-2)|\,\,unit^2\)
\(=\frac{1}{2}|-48-10|\,\,unit^2\)
\(=\frac{1}{2}|-58|\,\,unit^2\)
\(=\frac{1}{2}×58\,\,unit^2\)
\(=29\,\,unit^2\)
Area of ΔACD
\(=\frac{1}{2}|-4(-5+)+5(-2-5)+5(-4)(5+5)|\, unit^2\)
\(=\frac{1}{2}|-4(3)+5(-7)-4|\,\,unit^2\)
\(=\frac{1}{2}|-12-35-40|\,\,unit^2\)
\(=\frac{1}{2}|-63|\,\,unit^2\)
\(=\frac{63}{2}\,\,unit^2\)
Thus, area (ABCD) \(=(29+\frac{29}{2})\,unit^2\,=\frac{58+63}{2}\,unit^2\,\frac{121}{2}\,unit^2\)
Top Questions on Slope of a line
- If \( 3^x = 9^{x-1} \), then the value of \( x \) is:
- TS POLYCET - 2025
- Mathematics
- Slope of a line
- A line passes through \( (-1, -3) \) and is perpendicular to \( x + 6y = 5 \). Its x-intercept is:
- KCET - 2025
- Mathematics
- Slope of a line
- If the slope of the line joining the points (4,2) and (3,-k) is -2, then the value of k is
- AP POLYCET - 2024
- Mathematics
- Slope of a line
- If points \( (5, 5) \), \( (10, k) \), and \( (-5, 1) \) are collinear, then the value of \( k \) is:
- JEECUP - 2024
- Mathematics
- Slope of a line
- Variance of 6, 7, 8, 9 is
- KEAM - 2024
- Mathematics
- Slope of a line
Questions Asked in CBSE Class XI exam
- Take one flower of the family Solanaceae and write its semi-technical description. Also draw their floral diagram.
- CBSE Class XI
- The Flower
- \(\text{tan x}=-\frac{4}{3},\text{x\, in\, quadrant \,II.}\)
- CBSE Class XI
- Trigonometric Functions of Sum and Difference of Two Angles
- Find \((x + 1)^6 - (x - 1)^6\). Hence or otherwise evaluate \((\sqrt2 + 1)^6 + (\sqrt2 - 1)^6.\)
- CBSE Class XI
- Binomial Theorem for Positive Integral Indices
- Find \((a + b)^4 - (a - b)^4\). Hence, evaluate \((\sqrt3 + \sqrt2)^4 - (\sqrt3 - \sqrt2)^4\).
- CBSE Class XI
- Binomial Theorem for Positive Integral Indices
- Find the derivative of
(i) 2x - \(\frac{3}{4}\)
(ii)(5x3+3x-1)(x-1)
(iii) x-3(5+3x) (iv)x5(3-6x-9)
(v) x-4(3-4x-5) (vi)\(\frac{2}{x+1}-\frac{x^2}{3x-1}\)- CBSE Class XI
- Derivatives
Concepts Used:
Straight lines
A straight line is a line having the shortest distance between two points.
A straight line can be represented as an equation in various forms, as show in the image below:
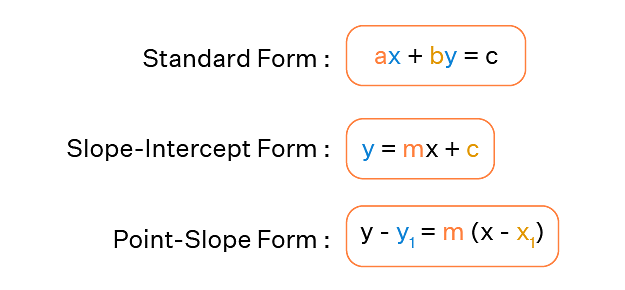
The following are the many forms of the equation of the line that are presented in straight line-
1. Slope – Point Form
Assume P0(x0, y0) is a fixed point on a non-vertical line L with m as its slope. If P (x, y) is an arbitrary point on L, then the point (x, y) lies on the line with slope m through the fixed point (x0, y0) if and only if its coordinates fulfil the equation below.
y – y0 = m (x – x0)
2. Two – Point Form
Let's look at the line. L crosses between two places. P1(x1, y1) and P2(x2, y2) are general points on L, while P (x, y) is a general point on L. As a result, the three points P1, P2, and P are collinear, and it becomes
The slope of P2P = The slope of P1P2 , i.e.
\(\frac{y-y_1}{x-x_1} = \frac{y_2-y_1}{x_2-x_1}\)
Hence, the equation becomes:
y - y1 =\( \frac{y_2-y_1}{x_2-x_1} (x-x1)\)
3. Slope-Intercept Form
Assume that a line L with slope m intersects the y-axis at a distance c from the origin, and that the distance c is referred to as the line L's y-intercept. As a result, the coordinates of the spot on the y-axis where the line intersects are (0, c). As a result, the slope of the line L is m, and it passes through a fixed point (0, c). The equation of the line L thus obtained from the slope – point form is given by
y – c =m( x - 0 )
As a result, the point (x, y) on the line with slope m and y-intercept c lies on the line, if and only if
y = m x +c