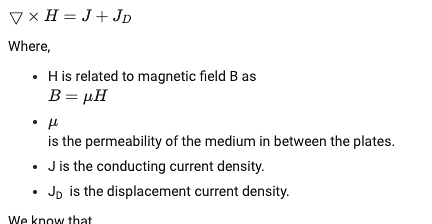
Question

Displacement current is caused due to

• A. a time varying electric filed
• B. a constant electric field
• C. free electrons flow
• D. all of the above

Answer 1

The Correct Option is A

As, displacement current,
$I_{d} = \varepsilon_{0} \frac{d\phi}{dt} \quad...\left(i\right) $
Where, $ \phi =$ electric flux
$\because \phi = E . A$
For area, $A =$ constant
$ \therefore d\phi = A \,dE \quad...\left(ii\right) $
From Eqs. $\left(i\right)$ and $\left(ii\right)$, we get $I_{d} = \varepsilon_{0}A \frac{dE}{dt} $
So, time varying electric field generates the displacement current