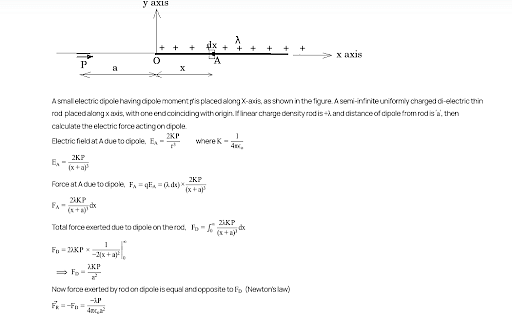
Question

Dipole placed in a sphere, what will be the electric flux?

Answer 1

If a dipole is placed inside a sphere, the electric flux through the sphere will depend on the orientation of the dipole with respect to the surface of the sphere.
The electric flux through a closed surface is given by Gauss's law, which states that the total electric flux through a closed surface is equal to the enclosed charge divided by the permittivity of the medium.
If the dipole moment is aligned with the normal vector of the surface, the electric flux through the sphere will be zero. This is because the positive and negative charges of the dipole cancel each other's contributions to the electric field at every point on the surface, resulting in no net flux passing through. If the dipole moment is not aligned with the normal vector of the surface, the electric flux through the sphere will be nonzero. In this case, the positive and negative charges of the dipole do not cancel each other completely, resulting in a net electric field passing through the surface of the sphere. This net electric field will lead to a nonzero electric flux through the sphere.
Therefore, the electric flux through the sphere with a dipole inside will depend on the orientation of the dipole relative to the surface of the sphere.