Question

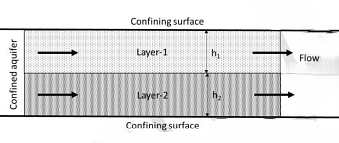
Consider two horizontal layers of an aquifer as shown in figure. Each layer is isotropic and homogeneous. Flow is parallel to the stratification. Thickness and horizontal hydraulic conductivity of layer-1 are $h_1$i and $K_1$, respectively. Thickness and horizontal hydraulic conductivity of layer-2 are $h_2$ and $K_2$, respectively, where $h_1$ is not equal to $h_2$. The equivalent horizontal conductivity $K_x$ for the aquifer system is given by _____

• A. \(k_x = \frac{k_1.h_1 + k_2.h_2}{h_1 + h_2}\)
• B. \(k_x = \frac{k_1+K_2}2\)
• C. \(k_x = \frac{k_1.h_3 + k_2.h_2}{h_1 + h_2}\)
• D. \(k_x = \sqrt{k_1.k_2}\)

Answer 1

The Correct Option is A

Step 1: Equivalent horizontal hydraulic conductivity. When flow is parallel to the stratification, the equivalent horizontal hydraulic conductivity \( K_x \) for the aquifer system is given by: \[ K_x = \frac{\sum (K_i \cdot h_i)}{\sum h_i} \] where \( K_i \) and \( h_i \) are the hydraulic conductivity and thickness of the \( i \)-th layer, respectively. 

Step 2: Substitute for two layers. For two layers, the equation becomes: \[ K_x = \frac{K_1 h_1 + K_2 h_2}{h_1 + h_2} \] 

Step 3: Interpretation of the terms. \( K_1 h_1 \): Contribution of layer-1 to the equivalent hydraulic conductivity. \( K_2 h_2 \): Contribution of layer-2 to the equivalent hydraulic conductivity. \( h_1 + h_2 \): Total thickness of the aquifer system. 

Step 4: Verify the given options. The correct formula for equivalent horizontal conductivity matches: \[ K_x = \frac{K_1 h_1 + K_2 h_2}{h_1 + h_2} \]