: At high pressure, the compression factor $Z$ is $\left(1+\frac{P b}{R T}\right)$.
: At high pressure van der Waals' equation is modified as $P(V-b)=R T$.
- If both Assertion and Reason are true and Reason is the correct explanation of Assertion.
- If both Assertion and Reason are true but Reason is not the correct explanation of Assertion.
- If Assertion is true but Reason is false.
- If both Assertion and Reason are false.
The Correct Option is A
Solution and Explanation
Top Questions on Van Der Waals equation
- Which of the following statemnt is incorect for physisorption?
- GUJCET - 2023
- Chemistry
- Van Der Waals equation
- Arrange the following gases in increasing order of van der Waals constant 'a'
A. Ar
B. CH4
C. H₂O
D. C6H6
Choose the correct option from the following.- JEE Main - 2023
- Chemistry
- Van Der Waals equation
- At low pressure, the van der Waal's equation is reduced to
- VITEEE - 2019
- Chemistry
- Van Der Waals equation
- Given van der Waals constant for NH3, H2, O2 and CO2 are respectively 4.17, 0.244, 1.36 and 3.59, which one of the following gases is most easily liquefied ?
- NEET (UG) - 2018
- Chemistry
- Van Der Waals equation
- If $V$ is the volume of one molecule of gas under given conditions, the van der Waal?s constant $b$ is
- BITSAT - 2018
- Chemistry
- Van Der Waals equation
Questions Asked in AIIMS exam
Given below are two statements, one is labelled as Assertion A and the other is labelled as Reason R
Assertion (A) : 02 is liberated in the non-cyclic photophosphorylation.
Reason (R) : Liberation of oxygen is due to photolysis of water.
In the light of the above statements, choose the correct answer from the options given below
- AIIMS - 2019
- Photosynthesis in higher plants
- Match the following column I with column II.
- AIIMS - 2019
- cloning
Given below are two statements, one is labelled as Assertion A and the other is labelled as Reason R
Assertion (A) : The Cro-Magnon man was the direct ancestor of the living modern man.
Reason (R) : Cro-Magnon man had slightly prognathous face.
In the light of the above statements, choose the correct answer from the options given below
- AIIMS - 2019
- evolution
- Given below are two statements, one is labelled as Assertion A and the other is labelled as Reason R
Assertion: Orbit of a satellite is within the gravitational field of earth whereas escaping is beyond the gravitational field of earth.
Reason: Orbital velocity of a satellite is greater than its escape velocity.
In the light of the above statements, choose the correct answer from the options given below- AIIMS - 2019
- Gravitation
Given below are two statements, one is labelled as Assertion A and the other is labelled as Reason R
Assertion (A) : In eukaryotes, transcription occurs in nucleus.
Reason (R) : In bacteria, transcription and translation occurs in cytoplasm.
In the light of the above statements, choose the correct answer from the options given below
- AIIMS - 2019
- Transcription
Concepts Used:
Van Der Waals Equation
Van der Waals equation is an equation relating the relationship between the pressure, volume, temperature, and amount of real gases.
Read More: Derivation of Van Der Waals Equation
Derivation of Van der Waals equation:
For a real gas containing ‘n’ moles, the equation is written as
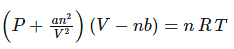
Where, P, V, T, n are the pressure, volume, temperature and moles of the gas. ‘a’ and ‘b’ constants specific to each gas.
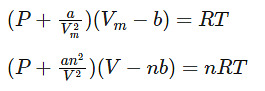
Where,
Vm: molar volume of the gas
R: universal gas constant
T: temperature
P: pressure
V: volume
Thus, Van der Waals equation can be reduced to ideal gas law as PVm = RT.
The equation can further be written as;
- Cube power of volume:
- Reduced equation (Law of corresponding states) in terms of critical constants:
Units of Van der Waals equation Constants
a: atm lit² mol-²
b: litre mol-¹