Question:

Area of triangle formed by the lines x+y=3 x + y = 3 and angle bisectors of the pair of straight lines x2−y2+2y=1x^2 - y^2 + 2y = 1 is

Updated On: Jun 14, 2022
  • 2 sq units
  • 4 sq units
  • 6 sq units
  • 8 sq units
Hide Solution
collegedunia
Verified By Collegedunia

The Correct Option is A

Solution and Explanation

Given, x2−y2+2y=1 x^2 - y^2 + 2y = 1
⇒x2=(y−1)2\Rightarrow x^2 = ( y - 1)^2
⇒x=y−1\Rightarrow x = y - 1
and x = - y + 1

From the graph, it is clear that equation of angle bisectors are y=1y = 1 and x=0x = 0
∴\therefore Area of region bounded b y x + y = 3 ,x = 0 and y = 1 is
△=12×2×2=2 \triangle = \frac{1}{2} \times 2 \times 2 = 2 sq units.
Was this answer helpful?
0
0

Top Questions on Straight lines

View More Questions

Questions Asked in JEE Advanced exam

View More Questions

Concepts Used:

Straight lines

A straight line is a line having the shortest distance between two points. 

A straight line can be represented as an equation in various forms,  as show in the image below:

 

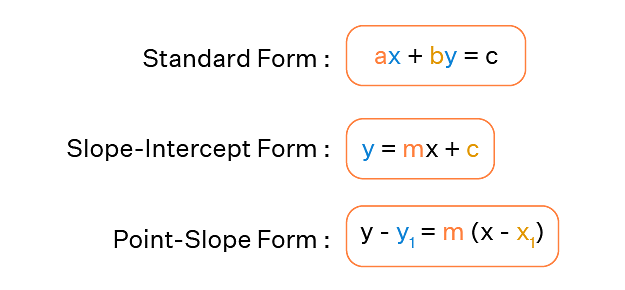
The following are the many forms of the equation of the line that are presented in straight line-

1. Slope – Point Form

Assume P0(x0, y0) is a fixed point on a non-vertical line L with m as its slope. If P (x, y) is an arbitrary point on L, then the point (x, y) lies on the line with slope m through the fixed point (x0, y0) if and only if its coordinates fulfil the equation below.

y – y0 = m (x – x0)

2. Two – Point Form

Let's look at the line. L crosses between two places. P1(x1, y1) and P2(x2, y2)  are general points on L, while P (x, y) is a general point on L. As a result, the three points P1, P2, and P are collinear, and it becomes

The slope of P2P = The slope of P1P2 , i.e.

y−y1x−x1=y2−y1x2−x1\frac{y-y_1}{x-x_1} = \frac{y_2-y_1}{x_2-x_1}

Hence, the equation becomes:

y - y1 =y2−y1x2−x1(x−x1) \frac{y_2-y_1}{x_2-x_1} (x-x1)

3. Slope-Intercept Form

Assume that a line L with slope m intersects the y-axis at a distance c from the origin, and that the distance c is referred to as the line L's y-intercept. As a result, the coordinates of the spot on the y-axis where the line intersects are (0, c). As a result, the slope of the line L is m, and it passes through a fixed point (0, c). The equation of the line L thus obtained from the slope – point form is given by

y – c =m( x - 0 )

As a result, the point (x, y) on the line with slope m and y-intercept c lies on the line, if and only if

y = m x +c