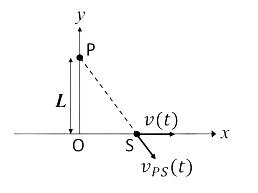
A whistle S of sound frequency f is oscillating with angular frequency 𝜔 along the x-axis. Its instantaneous position and the velocity are given by 𝑥(𝑡) = 𝑎 sin(𝜔𝑡) and 𝑣(𝑡)=𝑣0 cos (𝜔𝑡), respectively. An observer P is located on the y-axis at a distance L from the origin (see figure). Let 𝑣𝑃𝑆(𝑡) be the component of 𝑣(𝑡) along the line joining the source and the observer. Choose the correct option(s):
(Here 𝑎 and 𝑣0 are constants)
(Here 𝑎 and 𝑣0 are constants)

- 𝑣𝑃𝑆(𝑡)=\(\frac{1}{2}\frac{av_o}{\sqrt{a^2-sin^2wt+L^2}}sin(2wt)\)
- The observed frequency will be f when the source is at 𝑥 = 0 and 𝑥 = ±a
- The observed frequency will be f when the source is at position 𝑥 = ± \(\frac{a}{2}\)
- 𝑣𝑃𝑆(𝑡)=\(\frac{1}{2}\frac{av_o}{\sqrt{a^2+L^2}}sin(2wt)\)
The Correct Option is A, B
Solution and Explanation
Velocity Component Along the Line of Sight
The velocity component vPS(t) along the line joining the source and the observer is given by:
vPS(t) = v(t) cosϕ
where ϕ is the angle between the velocity vector and the line joining S and P.
Distance Between the Source and Observer
From the geometry of the problem, the distance between the source and the observer is:
r(t) = √(x(t)² + L²) = √(a² sin²(ωt) + L²)
The angle ϕ satisfies:
cosϕ = L / r(t)
Velocity Component Substitution
Substituting v(t) = v0 cos(ωt) and cosϕ = L / r(t), we have:
vPS(t) = v0 cos(ωt) × (L / √(a² sin²(ωt) + L²))
Using Trigonometric Identities
The component along the line of sight becomes:
vPS(t) = (1/2) × (a v0 / √(a² sin²(ωt) + L²)) sin(2ωt)
Thus, statement (A) is correct.
Doppler Effect and Observed Frequency
The observed frequency is given by the Doppler effect, which depends on the relative velocity component vPS(t).
- At x = 0 (midpoint of oscillation) and x = ±a (extremes of oscillation), the relative velocity component becomes zero, and the observed frequency equals the original frequency f.
Hence, statement (B) is correct.
Analyzing Other Statements
- At x = ±a/2, the velocity component vPS(t) is nonzero, so the observed frequency differs from f.
- Statement (D) is incorrect because vPS(t) depends on the varying distance r(t), not just √(a² + L²).
Conclusion
The correct statements are (A) and (B).
Top Questions on Waves and Oscillations
In an oscillating spring mass system, a spring is connected to a box filled with sand. As the box oscillates, sand leaks slowly out of the box vertically so that the average frequency ω(t) and average amplitude A(t) of the system change with time t. Which one of the following options schematically depicts these changes correctly?

- NEET (UG) - 2025
- Physics
- Waves and Oscillations
- Two identical point masses P and Q, suspended from two separate massless springs of spring constants \(k_1\) and \(k_2\), respectively, oscillate vertically. If their maximum velocities are the same, the ratio of the amplitude of P to the amplitude of Q is :
- NEET (UG) - 2025
- Physics
- Waves and Oscillations
- A simple pendulum has a length of $ L = 2 \, \text{m} $. What is the time period of the pendulum? (Assume $ g = 9.8 \, \text{m/s}^2 $)
- MHT CET - 2025
- Physics
- Waves and Oscillations
- Two tuning forks produce 6 beats per second when sounded together. One of the fork is in unison with 1.5 m length of wire and the other with 2.0 m length of wire. The frequencies of forks are:
- CUET (PG) - 2025
- Geophysics
- Waves and Oscillations
- A wave of frequency 500 Hz is travelling with a velocity 1000 m/s. How far are two points situated in wave whose displacement differ in phase by \( \frac{\pi}{3} \)?
- CUET (PG) - 2025
- Geophysics
- Waves and Oscillations
Questions Asked in IIT JAM exam
- The shortest distance between an object and its real image formed by a thin convex lens of focal length 20 cm is _____ cm. (in integer)
- If \(\left(\frac{1-i}{1+i}\right)^{n/2} = -1\), where \(i = \sqrt{-1}\), one possible value of n is
- IIT JAM PHY - 2025
- Complex numbers
- In a two-level atomic system, the excited state is 0.2 eV above the ground state. Considering the Maxwell-Boltzmann distribution, the temperature at which 2% of the atoms will be in the excited state is _____ K. (up to two decimal places)
(Boltzmann constant \(k_B = 8.62 \times 10^{-5}\) eV/K)
- IIT JAM PHY - 2025
- Mechanics
At a particular temperature T, Planck's energy density of black body radiation in terms of frequency is \(\rho_T(\nu) = 8 \times 10^{-18} \text{ J/m}^3 \text{ Hz}^{-1}\) at \(\nu = 3 \times 10^{14}\) Hz. Then Planck's energy density \(\rho_T(\lambda)\) at the corresponding wavelength (\(\lambda\)) has the value \rule{1cm}{0.15mm} \(\times 10^2 \text{ J/m}^4\). (in integer)
[Speed of light \(c = 3 \times 10^8\) m/s]
(Note: The unit for \(\rho_T(\nu)\) in the original problem was given as J/m³, which is dimensionally incorrect for a spectral density. The correct unit J/(m³·Hz) or J·s/m³ is used here for the solution.)- IIT JAM PHY - 2025
- Mechanics
- The ratio of the density of atoms between the (111) and (110) planes in a simple cubic (sc) lattice is ____. (up to two decimal places)
- IIT JAM PHY - 2025
- Solid State Physics, Devices, Electronics