Question

A transistor (β=100, 𝑉𝐵𝐸 = 0.7𝑉) is connected as shown in the circuit below.

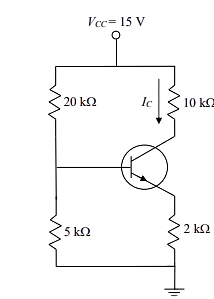
The current IC will be mA. (Rounded off to two decimal places)

Answer 1

Correct Answer: 1.1

Steps to Calculate \( I_C \) 

Step 1: Voltage at the Base (\( V_B \))

The base voltage \( V_B \) is determined by the voltage divider formed by the 20kΩ and 5kΩ resistors:

\[ V_B = V_{CC} \cdot \frac{5k\Omega}{20k\Omega + 5k\Omega} \]

Substituting \( V_{CC} = 15V \):

\[ V_B = 15 \cdot \frac{5}{25} \]

\[ V_B = 3.0V \]

Step 2: Voltage at the Emitter (\( V_E \))

The emitter voltage \( V_E \) is related to the base voltage by:

\[ V_E = V_B - V_{BE} \]

Substituting \( V_{BE} = 0.7V \):

\[ V_E = 3.0 - 0.7 = 2.3V \]

Step 3: Emitter Current (\( I_E \))

The emitter current \( I_E \) is determined by the 2kΩ resistor:

\[ I_E = \frac{V_E}{2k\Omega} \]

Substituting \( V_E = 2.3V \):

\[ I_E = \frac{2.3}{2000} \]

\[ I_E = 1.15mA \]

Step 4: Collector Current (\( I_C \))

The collector current \( I_C \) is related to the emitter current \( I_E \) by:

\[ I_C = \frac{\beta}{\beta + 1} I_E \]

Substituting \( \beta = 100 \):

\[ I_C = \frac{100}{101} \times 1.15 \]

\[ I_C \approx 1.14mA \]

Final Answer

Thus, the collector current \( I_C \) is approximately 1.14mA.