Question

A thermodynamic system is taken through the cycle abcda. The work done by the gas along the path bc is :

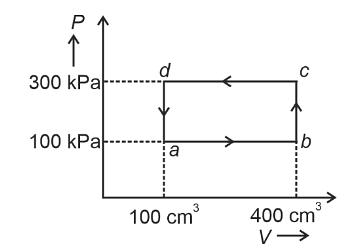
• A. Zero
• B. 30 J
• C. –90 J
• D. –60 J

Answer 1

The Correct Option is A

Understanding the Concept

Work done by a gas in a thermodynamic process is given by the integral of pressure with respect to volume:

\[ W = \int P \, dV \]

In simpler terms, on a P-V diagram, the work done is represented by the area under the curve for the given process.

Analyzing Path bc

Looking at the P-V diagram, path bc is a vertical line. This means the volume remains constant during this process. Such a process is called an isochoric or isovolumetric process.

Since the volume doesn't change (dV = 0), the work done along this path is:

\[ W = \int P \, dV = 0 \]

Therefore, the work done by the gas along the path bc is 0.