A spherical surface of radius of curvature R, separates air (refractive index 1.0) from glass (refractive index 1.5). The centre of curvature is in the glass. A point object P placed in air is found to have a real image Q in the glass. The line PQ cuts the surface at a point O and PO = O The distance PO is equal to
- 5 R
- 3 R
- 2 R
- 1.5 R
The Correct Option is A
Solution and Explanation
The correct option is(A): 5 R.
Let us say PO = OQ = X
Applying\(\, \, \, \, \, \, \, \, \frac{\mu_2}{v}-\frac{\mu_1}{u}=\frac{\mu_1-\mu_2}{R}\)
Substituting the values with sign
\(\frac{1.5}{+X}-\frac{1.0}{-X}=\frac{1.5-1.0}{+R}\)
(Distances are measured from O and are taken as positive in
the direction of ray of light)
\(\therefore\hspace15mm \frac{2.5}{X}=\frac{0.5}{R}\)
\(\therefore\hspace15mm X=5R\)
Top Questions on Spherical Mirrors
- A concave mirror produces an image of an object such that the distance between the object and image is 20 cm. If the magnification of the image is \( -3 \), then the magnitude of the radius of curvature of the mirror is:
- JEE Main - 2025
- Physics
- Spherical Mirrors
- (ii) An object at a distance of 16 cm from a spherical mirror forms a virtual image at a distance of 12 cm behind the mirror. Determine the magnification of the image and type of the mirror.
- UP Board X - 2025
- Science
- Spherical Mirrors
- Image of an object formed by a concave mirror is real and of the size of the object. The object is placed -
- UP Board X - 2025
- Science
- Spherical Mirrors
- With the help of a suitable ray diagram, derive the formula \( \frac{1}{v} + \frac{1}{u} = \frac{1}{f} \) for a concave mirror.
- UP Board XII - 2025
- Physics
- Spherical Mirrors
- The length of the image formed by a concave mirror:
- UP Board XII - 2025
- Physics
- Spherical Mirrors
Questions Asked in NEET exam
The output (Y) of the given logic implementation is similar to the output of an/a …………. gate.

- NEET (UG) - 2025
- Logic gates
- Two identical point masses P and Q, suspended from two separate massless springs of spring constants \(k_1\) and \(k_2\), respectively, oscillate vertically. If their maximum velocities are the same, the ratio of the amplitude of P to the amplitude of Q is :
- NEET (UG) - 2025
- Waves and Oscillations
- A particle of mass \(m\) is moving around the origin with a constant speed \(v\) along a circular path of radius \(R\). When the particle is at \( (0, R) \), its velocity is \( \mathbf{v} = -v \hat{\mathbf{i}} \). The angular momentum of the particle with respect to the origin is :
- NEET (UG) - 2025
- The Angular Momentum
- The radius of Martian orbit around the Sun is about 1.5 times the radius of the orbit of Mercury. The Martian year is 687 Earth days. Then which of the following is the length of 1 year on Mercury?
- NEET (UG) - 2025
- Kepler’s laws
- A balloon is made of a material of surface tension S and has a small outlet. It is filled with air of density \( \rho \). Initially the balloon is a sphere of radius R. When the gas is allowed to flow out slowly at a constant rate, its radius shrinks as \( r(t) \). Assume that the pressure inside the balloon is \( P(r) \) and is more than the outside pressure (\( P_0 \)) by an amount proportional to the surface tension and inversely proportional to the radius. The balloon bursts when its radius reaches \( r_0 \). Then the speed of gas coming out of the balloon at \( r = R \) is :
- NEET (UG) - 2025
- Surface Tension
Concepts Used:
Spherical Mirrors
A spherical mirror is a mirror which has been cut out of a spherical surface.
There are two kinds of spherical mirrors:
- Convex Mirror
- Concave Mirror
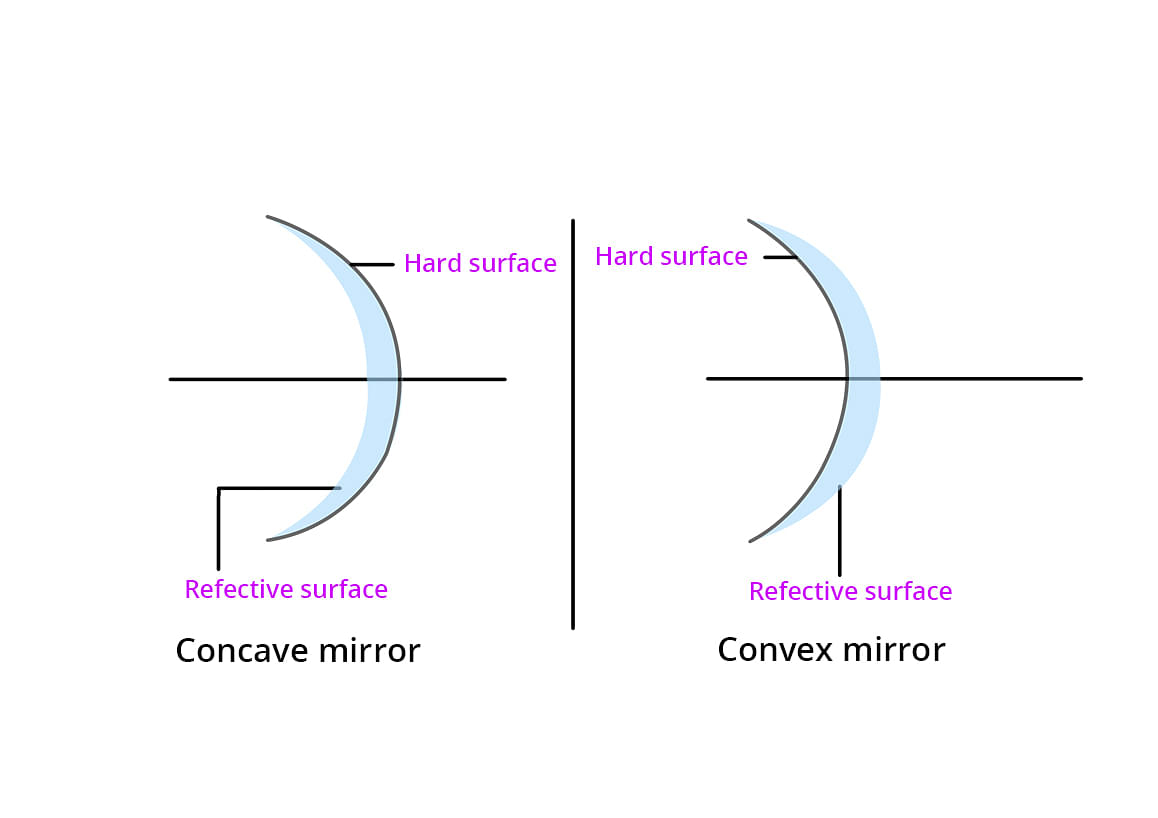
Concave Mirror
Concave mirrors are also called converging mirrors, because in these types of mirrors, light rays converge at a point after impact and reflect back from the reflective surface of the mirror.
Convex Mirror
The convex mirror has a reflective surface that is curved outward. Regardless of the distance between the subject and the mirrors, these mirrors are "always" virtual, upright and reduced.