A rigid bar of mass 15 kg is supported symmetrically by three wires each 2.0 m long. Those at each end are of copper and the middle one is of iron. Determine the ratios of their diameters if each is to have the same tension.
Solution and Explanation
The tension force acting on each wire is the same. Thus, the extension in each case is the same. Since the wires are of the same length, the strain will also be the same.
The relation for Young’s modulus is given as :
\(Y = \frac{Stress }{ Strain }= \frac{\frac{F }{ A} }{ Strain }= \frac{\frac{4F}{πd^2} }{ Strain} ....(i)\)
Where,
F = Tension force
A = Area of cross-section
d = Diameter of the wire
It can be inferred from equation (i) that \(Y ∝ \frac{1 }{ d^2}\)
Young’s modulus for iron, Y1 = 190 × 10 9 Pa
Diameter of the iron wire = d1
Young’s modulus for copper, Y2 = 110 × 10 9 Pa
Diameter of the copper wire = d2
Therefore, the ratio of their diameters is given as :
\(\frac{d_2 }{ d_1} = \frac{√Y_1 }{ Y_2} = \sqrt\frac{{190 × 10 ^9 }}{ 110 × 10^ 9 }= \sqrt\frac{19 }{11} \) = 1.31:1
Top Questions on elastic moduli
- The slope of the stress-strain curve in the elastic deformation region is
- JKCET - 2024
- Physics
- elastic moduli
- The law which states that within elastic limits strain produced is proportional to the stress producing it is known as
- JKCET - 2024
- Physics
- elastic moduli
- A wire of length L and radius r is clamped rigidly at one end. When the other end of the wire is pulled by a force F, its length increases by 5 cm. Another wire of the same material of length 4L and radius 4r is pulled by a force 4F under same conditions. The increase in length of this wire is ___ cm.
- JEE Main - 2022
- Physics
- elastic moduli
The elastic behavior of material for linear stress and linear strain, is shown in the figure. The energy density for a linear strain of 5×10–4 is ____ kJ/m3. Assume that material is elastic up to the linear strain of 5×10–4
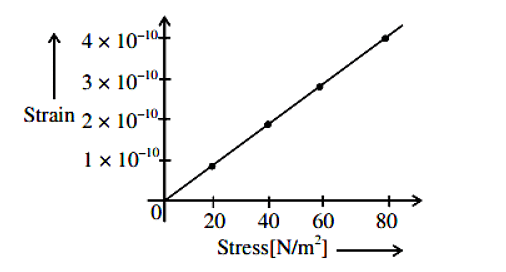
- JEE Main - 2022
- Physics
- elastic moduli
- If the length of a wire is made double and radius is halved of its respective values. Then, the Young’s modulus of the material of the wire will :
- JEE Main - 2022
- Physics
- elastic moduli
Questions Asked in CBSE Class XI exam
- Discuss the shape of the following molecules using the VSEPR model:
BeCl2, BCl3, SiCl4, AsF5, H2S, PH3- CBSE Class XI
- The Valence Shell Electron Pair Repulsion (VSEPR) Theory
- What do you understand by isoelectronic species? Name a species that will be isoelectronic with each of the following atoms or ions.
- F-
- Ar
- Mg2+
- Rb+
- CBSE Class XI
- Electronic Configurations Of Elements And The Periodic Table
- Take one flower of the family Solanaceae and write its semi-technical description. Also draw their floral diagram.
- CBSE Class XI
- The Flower
- \(\text{tan x}=-\frac{4}{3},\text{x\, in\, quadrant \,II.}\)
- CBSE Class XI
- Trigonometric Functions of Sum and Difference of Two Angles
- Find \((x + 1)^6 - (x - 1)^6\). Hence or otherwise evaluate \((\sqrt2 + 1)^6 + (\sqrt2 - 1)^6.\)
- CBSE Class XI
- Binomial Theorem for Positive Integral Indices