A 14.5 kg mass, fastened to the end of a steel wire of unstretched length 1.0 m, is whirled in a vertical circle with an angular velocity of 2 rev/s at the bottom of the circle. The cross-sectional area of the wire is 0.065 cm2 . Calculate the elongation of the wire when the mass is at the lowest point of its path.
Solution and Explanation
Mass, m = 14.5 kg
Length of the steel wire, l = 1.0 m
Angular velocity, ω = 2 rev / s
Cross-sectional area of the wire, a = 0.065 cm2
Let δl be the elongation of the wire when the mass is at the lowest point of its path. When the mass is placed at the position of the vertical circle, the total force on the mass is: F = mg + mlω2
= 14.5 × 9.8 + 14.5 × 1 × (2)2
= 200.1 N
\(Young's modulus = \frac{Stress }{ Strain}\)
\(Y =\frac{ \frac{F }{ A} }{ \frac{Δl }{ l} }= \frac{F \,l }{A Δl}\)
\(∴ Δl =\frac{ Fl }{ AY}\)
Young’s modulus for steel = 2 × 10 11 Pa
\(∴ Δl = \frac{200.1 × 1 }{ 0.065 × 10^{ - 4} × 2 × 10 ^{11}} = 1539.23 × 10 ^{- 7} \)
= 1.539 × 10 - 4 m
Hence, the elongation of the wire is 1.539 × 10 - 4 m.
Top Questions on elastic moduli
- The slope of the stress-strain curve in the elastic deformation region is
- JKCET - 2024
- Physics
- elastic moduli
- The law which states that within elastic limits strain produced is proportional to the stress producing it is known as
- JKCET - 2024
- Physics
- elastic moduli
- A wire of length L and radius r is clamped rigidly at one end. When the other end of the wire is pulled by a force F, its length increases by 5 cm. Another wire of the same material of length 4L and radius 4r is pulled by a force 4F under same conditions. The increase in length of this wire is ___ cm.
- JEE Main - 2022
- Physics
- elastic moduli
The elastic behavior of material for linear stress and linear strain, is shown in the figure. The energy density for a linear strain of 5×10–4 is ____ kJ/m3. Assume that material is elastic up to the linear strain of 5×10–4
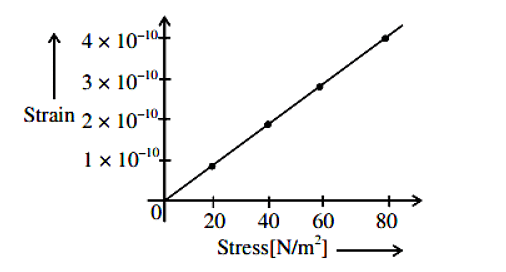
- JEE Main - 2022
- Physics
- elastic moduli
- If the length of a wire is made double and radius is halved of its respective values. Then, the Young’s modulus of the material of the wire will :
- JEE Main - 2022
- Physics
- elastic moduli
Questions Asked in CBSE Class XI exam
Give reasons for the following.
(i) King Tut’s body has been subjected to repeated scrutiny.
(ii) Howard Carter’s investigation was resented.
(iii) Carter had to chisel away the solidified resins to raise the king’s remains.
(iv) Tut’s body was buried along with gilded treasures.
(v) The boy king changed his name from Tutankhaten to Tutankhamun.- CBSE Class XI
- Discovering Tut: The saga Continues
- Write the atomic number of the element present in the third period and seventeenth group of the periodic table.
- CBSE Class XI
- Electronic Configurations Of Elements And The Periodic Table
- Discuss the shape of the following molecules using the VSEPR model:
BeCl2, BCl3, SiCl4, AsF5, H2S, PH3- CBSE Class XI
- The Valence Shell Electron Pair Repulsion (VSEPR) Theory
- What do you understand by isoelectronic species? Name a species that will be isoelectronic with each of the following atoms or ions.
- F-
- Ar
- Mg2+
- Rb+
- CBSE Class XI
- Electronic Configurations Of Elements And The Periodic Table
- Take one flower of the family Solanaceae and write its semi-technical description. Also draw their floral diagram.
- CBSE Class XI
- The Flower