The shortest distance between the line $y-x=1$ and the curve $x = y^2$ is
- $\frac{3\sqrt{2}}{8}$
- $\frac{2\sqrt{3}}{8}$
- $\frac{3\sqrt{2}}{5}$
- $\frac{\sqrt{3}}{4}$
The Correct Option is A
Solution and Explanation
Top Questions on Straight lines
- The equation of a straight line is given by \( y = 3x + 4 \). What is the slope of the line?
- BITSAT - 2025
- Mathematics
- Straight lines
- Find the slope of the line passing through the points $ (1, 2) $ and $ (3, 6) $:
- BITSAT - 2025
- Mathematics
- Straight lines
- The equation of the line passing through the point \((1, 2)\) and perpendicular to the line \(3x + 4y - 12 = 0\) is:
- AP EAPCET - 2025
- Mathematics
- Straight lines
- Find the slope of the line perpendicular to the line $ 3x + 4y - 12 = 0 $.
- AP EAPCET - 2025
- Mathematics
- Straight lines
- Two straight lines \( 3x - 2y = 5 \) and \( 2x + ky + 7 = 0 \) are perpendicular to each other. The value of \( k \) is:
- JEECUP - 2024
- Mathematics
- Straight lines
Questions Asked in AIEEE exam
- If the line $y = mx + 1$ meets the circle $x^2 + y^2 + 3x = 0 $ in two points equidistant from and on opposite sides of $x$-axis, then
- AIEEE - 2012
- Conic sections
- This question has Statement 1 and Statement 2. Of the four choices given after the Statements, choose the one that best describes the two Statements. If you push on a cart being pulled by a horse so that it does not move, the cart pushes you back with an equal and opposite force. The cart does not move because the force described in statement 1 cancel each other.
- AIEEE - 2012
- potential energy
- The variance of first n odd natural numbers is $\frac{n^{2}-1}{3}$ : The sum of first n odd natural number is $n^2$ and the sum of square of first n odd natural numbers is $\frac{n\left(4n^{2}-1\right)}{3}.$
- AIEEE - 2012
- Variance and Standard Deviation
- The ratio of number of oxygen atoms (O) in 16.0 g ozone $(O_3), \,28.0\, g$ carbon monoxide $(CO)$ and $16.0$ oxygen $(O_2)$ is (Atomic mass: $C = 12,0 = 16$ and Avogadro?? constant $N_A = 6.0 x 10^{23}\, mol^{-1}$)
- AIEEE - 2012
- Mole concept and Molar Masses
- This question has Statement 1 and Statement 2. Of the four choices given after the Statements, choose the one that best describes the two Statements. It is not possible to make a sphere of capacity $1$ farad using a conducting material. It is possible for earth as its radius is $6.4 \times 10^6\,m$.
- AIEEE - 2012
- electrostatic potential and capacitance
Concepts Used:
Straight lines
A straight line is a line having the shortest distance between two points.
A straight line can be represented as an equation in various forms, as show in the image below:
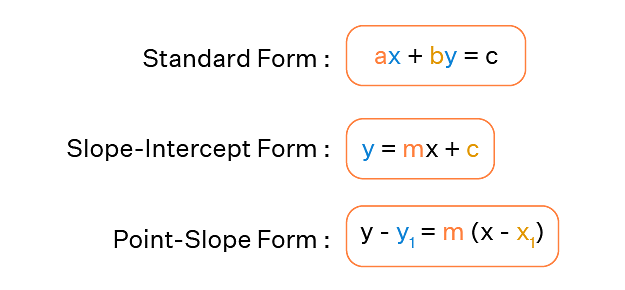
The following are the many forms of the equation of the line that are presented in straight line-
1. Slope – Point Form
Assume P0(x0, y0) is a fixed point on a non-vertical line L with m as its slope. If P (x, y) is an arbitrary point on L, then the point (x, y) lies on the line with slope m through the fixed point (x0, y0) if and only if its coordinates fulfil the equation below.
y – y0 = m (x – x0)
2. Two – Point Form
Let's look at the line. L crosses between two places. P1(x1, y1) and P2(x2, y2) are general points on L, while P (x, y) is a general point on L. As a result, the three points P1, P2, and P are collinear, and it becomes
The slope of P2P = The slope of P1P2 , i.e.
\(\frac{y-y_1}{x-x_1} = \frac{y_2-y_1}{x_2-x_1}\)
Hence, the equation becomes:
y - y1 =\( \frac{y_2-y_1}{x_2-x_1} (x-x1)\)
3. Slope-Intercept Form
Assume that a line L with slope m intersects the y-axis at a distance c from the origin, and that the distance c is referred to as the line L's y-intercept. As a result, the coordinates of the spot on the y-axis where the line intersects are (0, c). As a result, the slope of the line L is m, and it passes through a fixed point (0, c). The equation of the line L thus obtained from the slope – point form is given by
y – c =m( x - 0 )
As a result, the point (x, y) on the line with slope m and y-intercept c lies on the line, if and only if
y = m x +c