Prove that, cos 6 x = 32cos6 x-48cos4 x+18cos2 x -1.
Solution and Explanation
L.H.S. = cos 6x
= cos3 (2x)
= 4 cos3 2x - 3 cos 2x [cos 3A = 4 cos3 A - 3 cos A]
= 4 [(2 cos2 x-1)3 -3 (2 cos2 x-1) [cos 2 x = 2 cos2 x-1]
= 4 [(2 cos2 x)3 -(1)3 -3 (2 cos2 x) 2 + 3 (2 cos2 x)]-6cos2 x + 3
= 4 [8 cos6 x-1-12 cos4 x+6 cos2 x]-6 cos2 x+3
= 32 cos6 x- 4 - 48 cos4 x+24 cos2 x-6 cos2 x+3
= 32 cos6 x - 48 cos4 x+18 cos2 x - 1
= R.H.S.
Top Questions on Trigonometric Functions of Sum and Difference of Two Angles
- In the figure, the value of cosec A is
- TS POLYCET - 2021
- Mathematics
- Trigonometric Functions of Sum and Difference of Two Angles
- Prove that cos 4x =1-8 sin2 x cos2 x
- CBSE Class XI
- Mathematics
- Trigonometric Functions of Sum and Difference of Two Angles
- Prove that sin x+sin 3x+sin 5x+sin 7x=4 cos x cos 2x sin 4x.
- CBSE Class XI
- Mathematics
- Trigonometric Functions of Sum and Difference of Two Angles
- Prove that. \(2sin^2 \frac{π}{6}+cosec^2 \frac{7π}{6}–cos^2 \frac{π}{3}=\frac{3}{2}\)
- CBSE Class XI
- Mathematics
- Trigonometric Functions of Sum and Difference of Two Angles
Prove that. \(sin^2 \frac{π}{6}+cos^2 \frac{π}{3}–tan^2 \frac{π}{4}=-\frac{1}{2}\)
- CBSE Class XI
- Mathematics
- Trigonometric Functions of Sum and Difference of Two Angles
Questions Asked in CBSE Class XI exam
- Consider the reactions:
- H3PO2(aq) + 4 AgNO3(aq) + 2 H2O(l) \(\rightarrow\) H3PO4(aq) + 4Ag(s) + 4HNO3(aq)
- H3PO2(aq) + 2CuSO4(aq) + 2 H2O(l) \(\rightarrow\) H3PO4(aq) + 2Cu(s) + H2SO4(aq)
- C6H5CHO(l) + 2[Ag (NH3)2]+(aq) + 3OH- (aq) \(\rightarrow\) C6H5COO- (aq) + 2Ag(s) + 4NH3(aq) + 2 H2O(l)
- C6H5CHO(l) + 2Cu2+(aq) + 5OH-(aq) \(\rightarrow\) No change observed.
What inference do you draw about the behaviour of Ag+ and Cu2+ from these reactions?
- CBSE Class XI
- Redox Reactions In Terms Of Electron Transfer Reactions
- Find \((x + 1)^6 - (x - 1)^6\). Hence or otherwise evaluate \((\sqrt2 + 1)^6 + (\sqrt2 - 1)^6.\)
- CBSE Class XI
- Binomial Theorem for Positive Integral Indices
- If U = {a, b, c, d, e, f, g, h}, find the complements of the following sets:
(i) A = {a, b, c}
(ii) B = {d, e, f, g}
(iii) C = {a, c, e, g}
(iv) D = {f, g, h, a}- CBSE Class XI
- Complement of a Set
- Consider the following species: N3- , O2-, F-, Na+, Mg2+ and Al3+
- What is common in them?
- Arrange them in the order of increasing ionic radii.
- CBSE Class XI
- Modern Periodic Law And The Present Form Of The Periodic Table
- Find and write down structures of 10 interesting small molecular weight biomolecules. Find if there is any industry which manufactures the compounds by isolation. Find out who are the buyers.
- CBSE Class XI
- How To Analyse Chemical Composition?
Concepts Used:
Trigonometric Functions
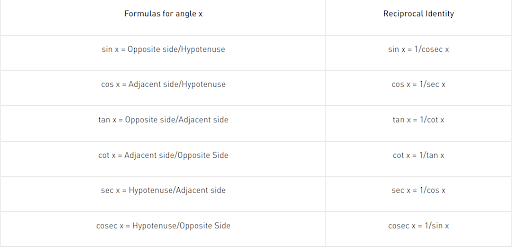
The relationship between the sides and angles of a right-angle triangle is described by trigonometry functions, sometimes known as circular functions. These trigonometric functions derive the relationship between the angles and sides of a triangle. In trigonometry, there are three primary functions of sine (sin), cosine (cos), tangent (tan). The other three main functions can be derived from the primary functions as cotangent (cot), secant (sec), and cosecant (cosec).
Six Basic Trigonometric Functions:
- Sine Function: The ratio between the length of the opposite side of the triangle to the length of the hypotenuse of the triangle.
sin x = a/h
- Cosine Function: The ratio between the length of the adjacent side of the triangle to the length of the hypotenuse of the triangle.
cos x = b/h
- Tangent Function: The ratio between the length of the opposite side of the triangle to the adjacent side length.
tan x = a/b
Tan x can also be represented as sin x/cos x
- Secant Function: The reciprocal of the cosine function.
sec x = 1/cosx = h/b
- Cosecant Function: The reciprocal of the sine function.
cosec x = 1/sinx = h/a
- Cotangent Function: The reciprocal of the tangent function.
cot x = 1/tan x = b/a
Formulas of Trigonometric Functions:
