If $ (3,\,\,3) $ is a vertex of a triangle and $ (-3,\,\,6) $ and $ (9,\,\,6) $ are the mid points of the two sides through this vertex, then the centroid of the triangle is
- $ (3,\,\,7) $
- $ (1,\,\,7) $
- $ (-3,\,\,7) $
- $ (-1,\,\,7) $
The Correct Option is A
Approach Solution - 1
Then, $ \frac{{{x}_{1}}+3}{2}=-3,\,\,\,\,\,\,\,\,\,\,\,\,\frac{{{y}_{1}}+3}{2}=6 $
$ \Rightarrow $ $ {{x}_{1}}=-9,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,{{y}_{1}}=9 $
and $ \frac{{{x}_{2}}+3}{2}=9,\,\,\,\,\,\,\,\,\,\,\,\,\,\frac{{{y}_{2}}+3}{2}=6 $
$ \Rightarrow $ $ {{x}_{2}}=15,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,{{y}_{2}}=9 $
Now, centroid $ =\left( \frac{-9+15+3}{3},\frac{9+9+3}{3} \right) $
$ =(3,7) $
Approach Solution -2
A triangle's centroid is the location inside the triangle where all of its medians meet or cross. Straight lines that connect a vertex to its opposite side and divide that side into two equal half are called medians. The triangle is divided into smaller, equal-sized triangles using a median as well. There are four locations when a triangle is concurrent.
Orthocentre
Incentre
Circumcentre
Centroid
A triangle's centroid is always found inside the triangle's perimeter.

The centroid of the triangle is shown by point G in this instance. The centroid of a triangle always resides inside the triangle's perimeter, just like the incentre does. To get the centroid's coordinates, the section formula of coordinate geometry is used. A triangle's centroid, which coincides with its medians, always rests on a median and splits it 2:1. The centroid theorem refers to this.
The relative midpoints of the triangle's sides are denoted by D, E, and F. The triangle ABC's centroid is labeled G.
Following is a discussion of the characteristics of a triangle's centroid:
- The point where all three of a triangle's medians meet or converge is known as the centroid.
- The medians are split in half at the centroid in a 2:1 ratio. The centroid theorem is another name for this.
- The most frequent point of concurrency inside a triangle is its centroid. Its significance in both mathematics and physics stems from the fact that it is the triangle's geometric core.
- The centroid's coordinates satisfy the straight-line equations for the three medians since it is the intersection of all three of them.
- It is always situated inside a triangle's rim.

The coordinates of the three vertices of the triangle ABC are A(x1, y1), B(x2, y2), and C(x3, y3). D, E, and F are the respective midpoints of the sides BC, AC, and AB. G is the centroid.
Then the coordinates of the centroid of the triangle ABC are given as :

Top Questions on Straight lines
- The equation of a straight line is given by \( y = 3x + 4 \). What is the slope of the line?
- BITSAT - 2025
- Mathematics
- Straight lines
- Find the slope of the line passing through the points $ (1, 2) $ and $ (3, 6) $:
- BITSAT - 2025
- Mathematics
- Straight lines
- The equation of the line passing through the point \((1, 2)\) and perpendicular to the line \(3x + 4y - 12 = 0\) is:
- AP EAPCET - 2025
- Mathematics
- Straight lines
- Find the slope of the line perpendicular to the line $ 3x + 4y - 12 = 0 $.
- AP EAPCET - 2025
- Mathematics
- Straight lines
- Two straight lines \( 3x - 2y = 5 \) and \( 2x + ky + 7 = 0 \) are perpendicular to each other. The value of \( k \) is:
- JEECUP - 2024
- Mathematics
- Straight lines
Questions Asked in JKCET exam
- 5 books in Math and 3 books in Physics are placed on a shelf so that the books on the same subject always remain together. The possible arrangements are
- JKCET - 2024
- Permutations
- If the length of a chord of a circle is equal to that of radius of the circle, then the angle subtended in radius, at the centre of the circle by the chord is
- Which of the following is a null set?
- Which of the following statement is true?
- Trans-esterification is a reaction between
- JKCET - 2024
- Organic Chemistry - Some Basic Principles and Techniques
Concepts Used:
Straight lines
A straight line is a line having the shortest distance between two points.
A straight line can be represented as an equation in various forms, as show in the image below:
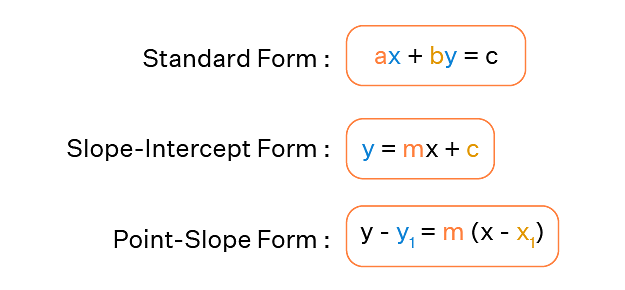
The following are the many forms of the equation of the line that are presented in straight line-
1. Slope – Point Form
Assume P0(x0, y0) is a fixed point on a non-vertical line L with m as its slope. If P (x, y) is an arbitrary point on L, then the point (x, y) lies on the line with slope m through the fixed point (x0, y0) if and only if its coordinates fulfil the equation below.
y – y0 = m (x – x0)
2. Two – Point Form
Let's look at the line. L crosses between two places. P1(x1, y1) and P2(x2, y2) are general points on L, while P (x, y) is a general point on L. As a result, the three points P1, P2, and P are collinear, and it becomes
The slope of P2P = The slope of P1P2 , i.e.
\(\frac{y-y_1}{x-x_1} = \frac{y_2-y_1}{x_2-x_1}\)
Hence, the equation becomes:
y - y1 =\( \frac{y_2-y_1}{x_2-x_1} (x-x1)\)
3. Slope-Intercept Form
Assume that a line L with slope m intersects the y-axis at a distance c from the origin, and that the distance c is referred to as the line L's y-intercept. As a result, the coordinates of the spot on the y-axis where the line intersects are (0, c). As a result, the slope of the line L is m, and it passes through a fixed point (0, c). The equation of the line L thus obtained from the slope – point form is given by
y – c =m( x - 0 )
As a result, the point (x, y) on the line with slope m and y-intercept c lies on the line, if and only if
y = m x +c