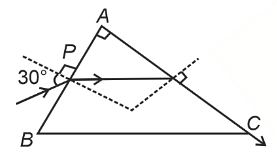
A light ray enters through a right angled prism at point P with the angle of incidence 30° as shown in figure. It travels through the prism parallel to its base BC and emerges along the face AC. The refractive index of the prism is :

- \(\frac{\sqrt5}{4}\)
- \(\frac{\sqrt5}{2}\)
- \(\frac{\sqrt3}{4}\)
- \(\frac{\sqrt3}{2}\)
The Correct Option is B
Solution and Explanation

Step 1: Relation in Prism
From the prism relation:
$$ r_1 + c = A $$
Rearranging for \( r_1 \):
$$ r_1 = 90^\circ - c \quad \text{...(1)} $$
Step 2: Expression for \( \cos c \)
We know:
$$ \sin c = \frac{1}{\mu} $$
Using trigonometric identity:
$$ \cos c = \frac{\sqrt{\mu^2 - 1}}{\mu} $$
Step 3: Apply Snell's Law on Incidence Surface
Using Snell’s law at the first surface:
$$ \sin 30^\circ = \mu \sin (r_1) $$
Substituting \( r_1 = 90^\circ - c \):
$$ \frac{1}{2} = \mu \sin (90^\circ - c) $$
Since \( \sin (90^\circ - c) = \cos c \), we get:
$$ \frac{1}{2} = \mu \times \frac{\sqrt{\mu^2 - 1}}{\mu} $$
Simplifying:
$$ \frac{1}{2} = \frac{\sqrt{\mu^2 - 1}}{1} $$
Step 4: Solve for \( \mu \)
Squaring both sides:
$$ \frac{1}{4} = \mu^2 - 1 $$
Rearranging:
$$ \mu^2 = \frac{5}{4} $$
Taking square root:
$$ \mu = \frac{\sqrt{5}}{2} $$
Conclusion
The refractive index \( \mu \) of the prism is \(\frac{\sqrt{5}}{2}\).
Top Questions on Refraction Through A Prism
- (ii) Show ray diagram for the refraction of a light ray through a prism and point out the emergent angle and angle of deviation.
- UP Board X - 2025
- Science
- Refraction Through A Prism
- Derive Lens Maker's formula for a thin lens. Mention the effect of the refractive index and radius of curvature of the curved surfaces on the focal length of the lens.
- UP Board XII - 2025
- Physics
- Refraction Through A Prism
- Prism angle of a prism is $A$ and angle of minimum deviation is equal to prism angle. Refractive index of the material of prism will be:
- UP Board XII - 2025
- Physics
- Refraction Through A Prism
- What are the conditions for the minimum deviation by a prism? One face (AC) of a prism of refracting angle 30° and refractive index \( \sqrt{2} \), is silvered. What should be the angle of incidence \( i \) on the surface AB, so that after refraction into the prism, the reflected ray from the silvered surface retraces its path? See the figure.
\includegraphics[width=0.5\linewidth]{image1.png}- UP Board XII - 2025
- Physics
- Refraction Through A Prism
- Draw a labelled ray diagram of an astronomical telescope and derive the formula of its magnifying power.
- UP Board XII - 2025
- Physics
- Refraction Through A Prism
Questions Asked in NEET exam
A bob of heavy mass \(m\) is suspended by a light string of length \(l\). The bob is given a horizontal velocity \(v_0\) as shown in figure. If the string gets slack at some point P making an angle \( \theta \) from the horizontal, the ratio of the speed \(v\) of the bob at point P to its initial speed \(v_0\) is :

- NEET (UG) - 2025
- Pendulums
- An oxygen cylinder of volume 30 litre has 18.20 moles of oxygen. After some oxygen is withdrawn from the cylinder, its gauge pressure drops to 11 atmospheric pressure at temperature \(27^\circ\)C. The mass of the oxygen withdrawn from the cylinder is nearly equal to: [Given, \(R = \frac{100}{12} \text{ J mol}^{-1} \text{K}^{-1}\), and molecular mass of \(O_2 = 32 \text{ g/mol}\), 1 atm pressure = \(1.01 \times 10^5 \text{ N/m}^2\)]
- NEET (UG) - 2025
- Ideal-gas equation and absolute temperature
- A uniform rod of mass 20 kg and length 5 m leans against a smooth vertical wall making an angle of \(60^\circ\) with it. The other end rests on a rough horizontal floor. The friction force that the floor exerts on the rod is (take \(g = 10\) m/s\(^2\)):
- NEET (UG) - 2025
- Common forces in mechanics
- A wire of resistance \(R\) is cut into 8 equal pieces. From these pieces, two equivalent resistances are made by adding four of these together in parallel. Then these two are added in series. The net effective resistance of the combination is:
- NEET (UG) - 2025
- Resistance
- Which of the following organisms cannot fix nitrogen? A. Azotobacter
B. Oscillatoria
C. Anabaena
D. Volvox
E. Nostoc
Choose the correct answer from the options given below:- NEET (UG) - 2025
- Nitrogen Fixation